Oncological hyperthermia: The correct dosing in clinical applications
- Authors:
- Published online on: November 23, 2018 https://doi.org/10.3892/ijo.2018.4645
- Pages: 627-643
-
Copyright : © Lee et al. This is an open access article distributed under the terms of Creative Commons Attribution License [CC BY 4.0].
Abstract
Introduction: Hyperthermia therapy in oncology
Hyperthermia is an ancient method; in fact, it was the very first medical treatment in human medicine. Despite its long history, this approach currently has no widespread applications and is on the periphery of the medical therapies. This contradiction characterizes the history of hyperthermia in medicine.
The use of hyperthermia as a therapy has various stumbling blocks as the effect caused by the absorbed heat is too complex; the applied, absorbed energy is usually depleted non-homogeneously, and the intricacy of biological processes modifies the intended impact of application. The heating process itself represents a further complication: The efficacy indeed differs by the heat source and by the properties of the target volume and its physiological effects. Hyperthermia treatment modalities have yet to progress from a bio-medical experiment to a clinically proven treatment (1,2).
The effect of hyperthermia is not straightforward in in vivo experiments and is even more complex in clinical practice. In clinical applications, hyperthermia has two essential effects: i) Thermal damage assessed by the Arrhenius approach and associated evaluations; and ii) physiology-dependent effects are improving the complementary applications.
While the simple thermal effect can be demonstrated using in vitro experiments, the evident clinical outcomes are not clearly observable. The challenge is the negative physiological feedback of systemic reactions, which tries to compensate for the increase in temperature and reassert thermal homeostasis.
Hyperthermia is a technically challenging treatment modality. Energy absorption during the heating process entirely depends on the technique applied, and there is no unified protocol for the various technologically determined targets of the heat. The ablation technique differs from the methods causing necrosis or apoptosis, or those that heat up the whole body into the fever range. The category 'hyperthermia' includes the group of energy-absorption methods, and the individual solutions of absorption require an actual protocol. It is very similar in this regard to the chemo-variants of oncological therapies. Chemotherapy, depending on the targets of the drug, has different protocols. Mixing these can cause severe adverse effects and even fatal events, such as poisoning. Homogeneous targeting in most of these therapies requires very different protocols than local or cell-sensitive selection. For example, chemotherapy is administered intravenously at different doses than with chemoembolization or other types of local administration. Isodose homogeneity, as in radiotherapy, is also not used in most of the brachytherapies, radiation seed or nanoparticle administration. We are sure that the hyperthermia variants also have specific differences in their dose and protocol, sharply depending on their technical solution and targeting method. Defining a general dose and protocol for all hyperthermia methods is a misleading request. The methods are not equal, their effects are different, and thus the dose and protocol have to fit the specific situation.
Oncological hyperthermia suffers presently multiple problems (3), among these the lack of underlying mechanisms of thermal therapies and the missing joint definition of the method and missing a well measurable dose for clinical and research applications.
The choice between thermal or non-thermal and between heat or temperature
The definition of oncological hyperthermia is not unified in the actual literature. The following are a few of the most common definitions found in the literature: i) Hyperthermia is the use of therapeutic heat to treat various types of cancer on and inside the body (4); ii) hyperthermia is the overheating of the body (5); iii) hyperthermia (also known as thermal therapy or thermotherapy) is a type of cancer treatment in which body tissue is exposed to high temperatures [up to 113°F (45°C)] (6); iv) hyperthermia therapy is a type of medical treatment in which body tissue is exposed to slightly higher temperatures to damage and kill cancer cells or to render cancer cells more sensitive to the effects of radiation and certain anti-cancer drugs (7); v) hyperthermia involves body temperature which is much higher than normal and is either induced therapeutically or iatrogenically (8); vi) hyperthermia occurs when cells in the body are exposed to temperatures which are higher than normal, and thus changes take place inside the cells (9).
The indefinite definition ranges in a broad spectrum of targets, heating the body, cancer or the malignant cells, and mixes the therapeutic heat and the therapeutic temperature. The key to thermotherapy is the amount and distribution of the absorbed energy in the malignant target. We prefer the definition of oncological hyperthermia as method with which to kill malignant cells by heat-inducing absorbed energy and/or sensitize specific complementary therapies.
The majority of the oncological hyperthermia methods work with absorbed energy by bio-electromagnetic interactions. The biological processes are fundamentally based on the only electromagnetic working ability. Bio-electromagnetic energy exchange is a broad category of energies in life, including all the chemical, mechanical, thermal and bio-electric changes energizing the living processes. The absorption of external energy is necessary for life, it forms an open system; it does not exist in an energetically closed (self-sustaining) mode.
Energy is a global category for all possible work processes. Heat is a type of energy, which can raise the temperature of the target, although not always. An increase in temperature is not the only effect in this complex interaction. The absorbed energy may be utilized for structural rearrangement or chemical reactions, or electromagnetic changes (such as polarization). The temperature strongly depends on how the heat is provided to the system, and how well the system is isolated from its environment. Heat can be transformed into complex changes in the target without an increase in temperature.
A simple example is boiling water. The water reaches its boiling point of 100°C, and its temperature from that time continually remains at 100°C; all the heat pumped in is used for evaporation (steam production). The energy is ultimately used to change the structure of the material from solid to liquid.
Temperature is not the heat or even energy of the system. It measures the average energy of the particles in a system. The average does not mean that every particle has the same energy in the equilibrium. The statistical average works differently. For example, the average yearly income in a country does not mean that everyone's salary is the same, and the average does not measure your level of earnings.
Absorbed energy differs from temperature, i.e., the average kinetic energy of particles. A good example is a comparison of warm water in a swimming pool and the same temperature of water in a glass. Heating up the pool needs much more energy than heating up a glass of water to the same temperature. Although heat and temperature are different, these are used equivalently in many instances. The main reason for confusing them is fixed attention to simple situations when the heat does not perform any ‘work’ in the system's internal energy; it is only homogeneously converted to the average, to the temperature. The energy averaging and characterizing the process by the temperature can happen when we heat clean water between its freezing and boiling points. However, this simple case is very far from the complexity of the living material, which is heterogeneous and uses absorbed heat energy for structural and chemical changes.
Using the temperature like a dose of heating is incorrect; dosing by temperature does not depend on the volume or mass of the target as it is an average and is the same in half or any fraction of the target, while the dose must be different for different sized targets. Due to this, heat is a good candidate for dosing.
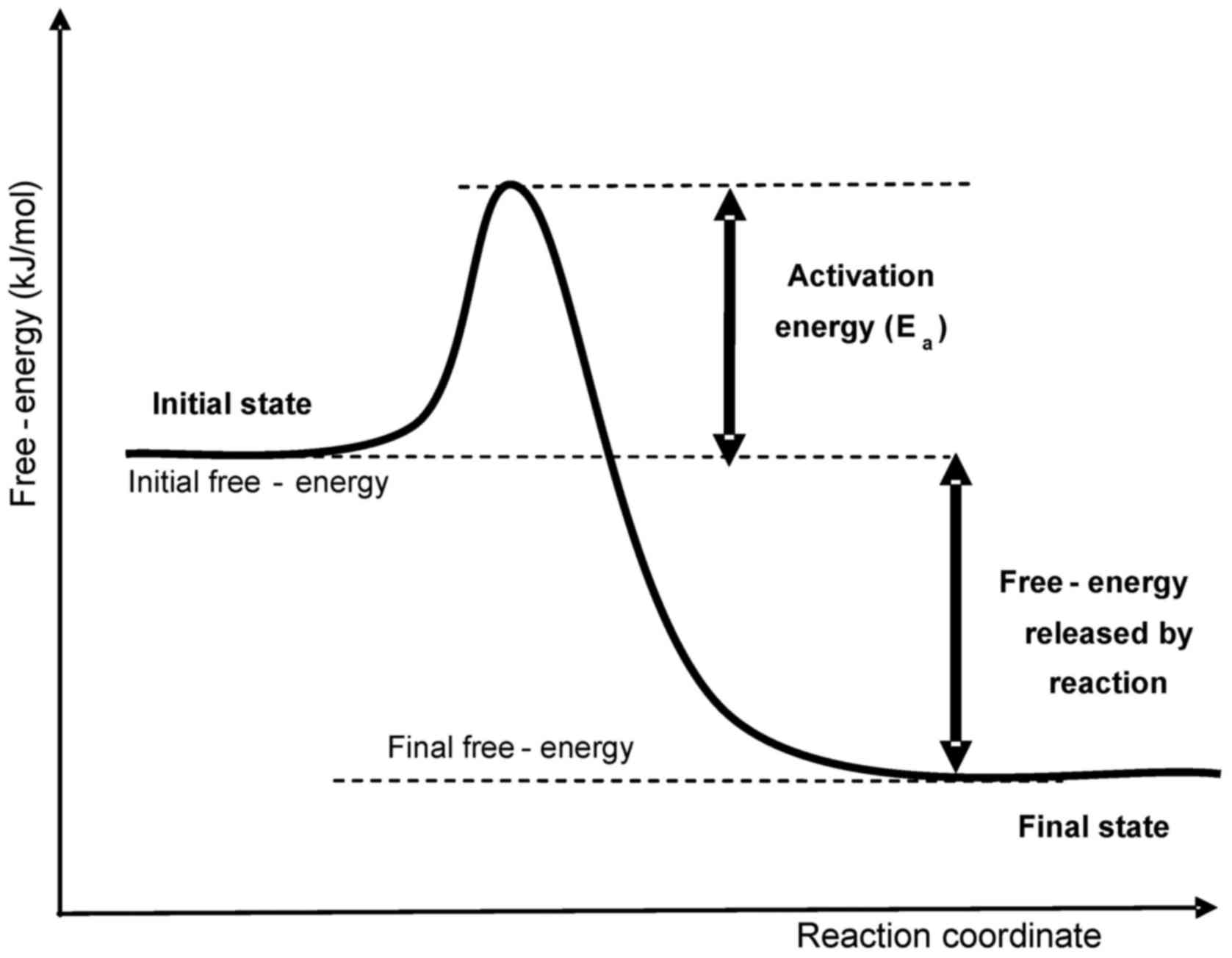
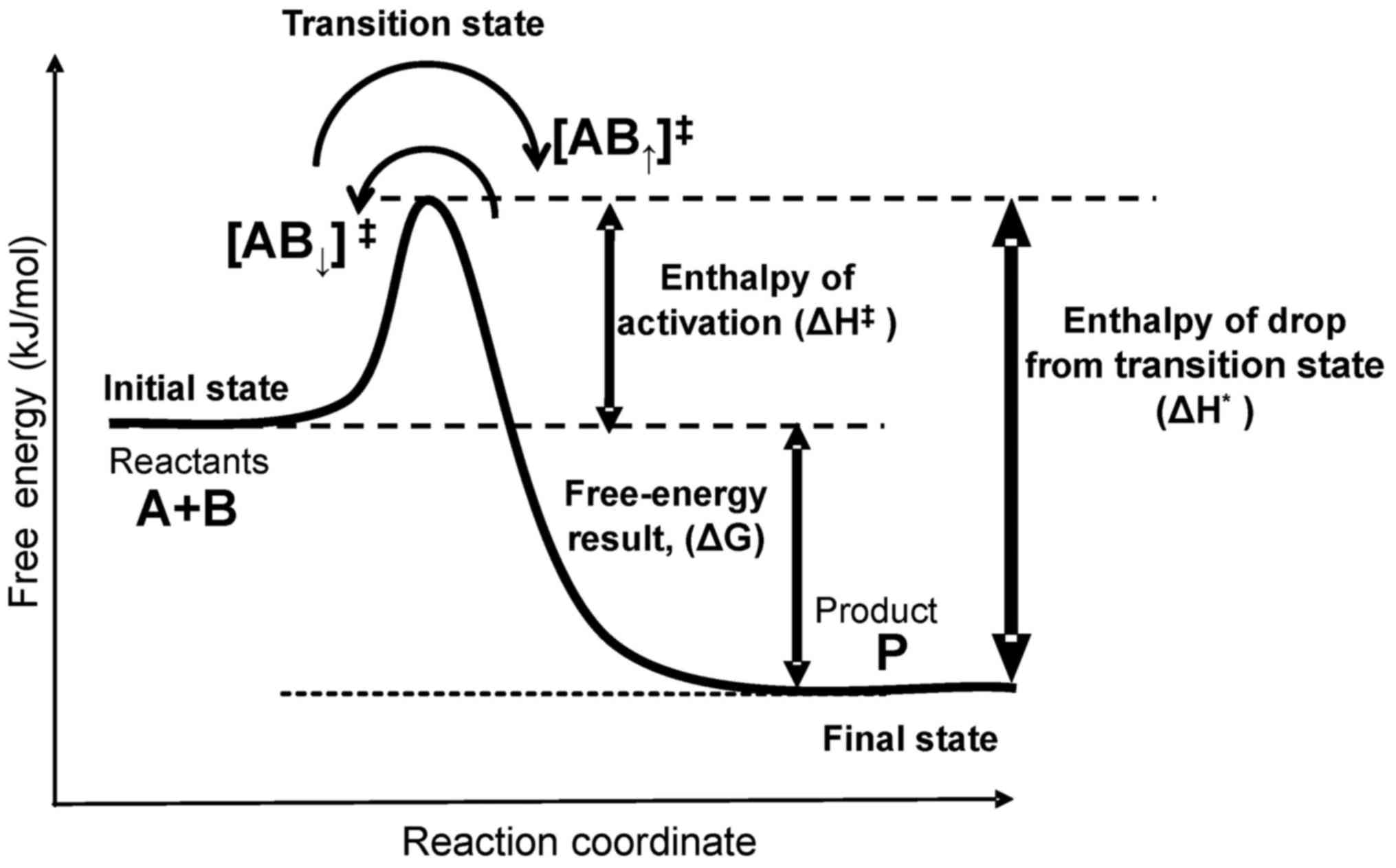
Keeping in mind the example of boiling water, holding the temperature at 100°C while pumping in a massive amount of energy to keep the water boiling is a thermal process, although the temperature does not change. The question thus automatically arises as to what is the proper characteristic that we define as ‘thermal’ when the temperature is not a stable indicator of a thermal process. The molecular mechanism of the thermal process defines the probability (reaction rate, kr) of a jump of a given particle through an energy barrier (activation energy, Ea, measured in J/mol) (Fig. 1).
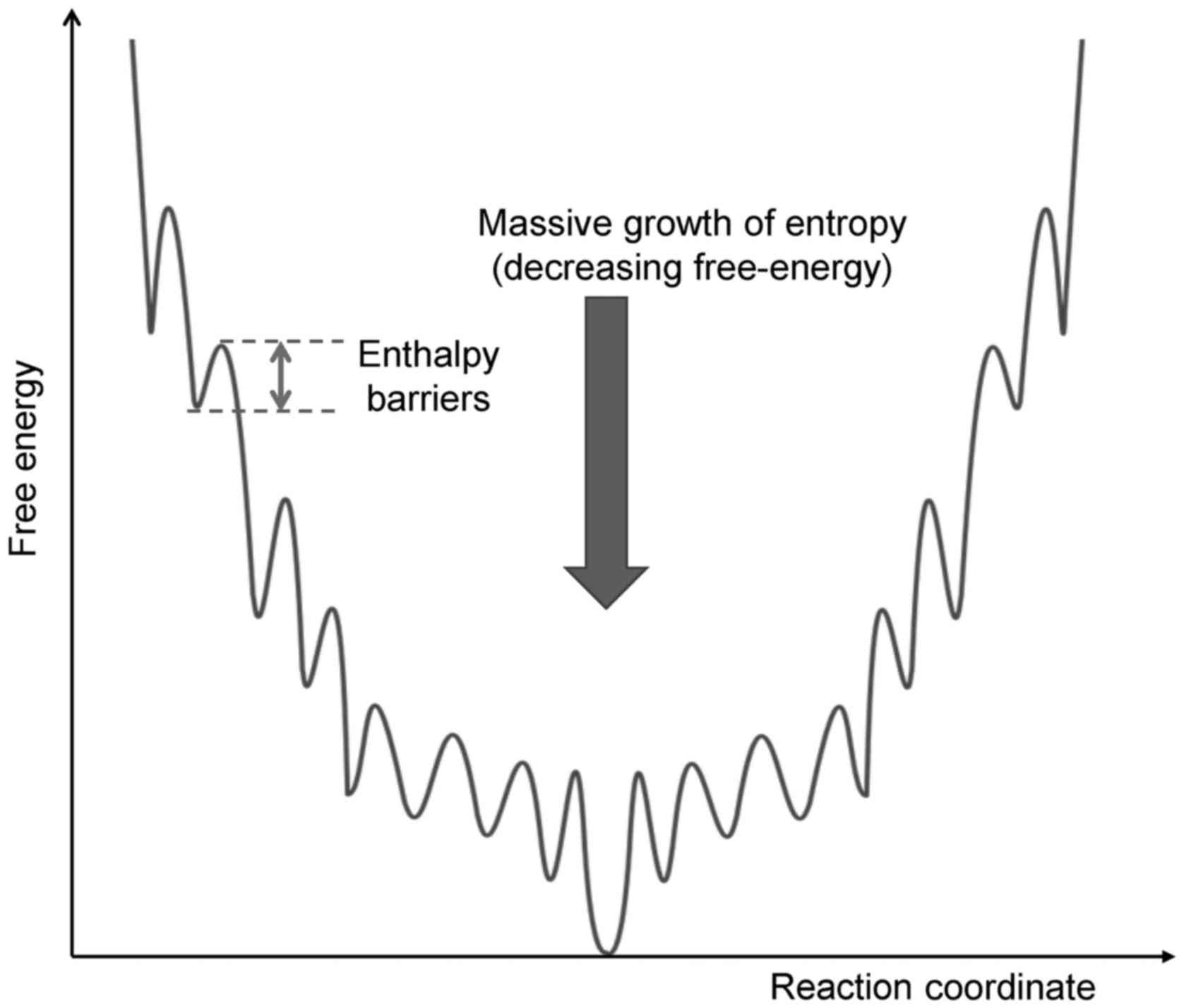
The energy barrier is mandatory for life processes; without it, an immediate reaction occurs, which explosively drives the reaction out of control. A living system has a funnel-type multibarrier system that gradually loses energy step-by-step during a jump through various reactions in a chain (Fig. 2).
Thermal processes are described by an empirical description of the reaction rate, i.e., the Arrhenius equation (10); [Equation 1]:
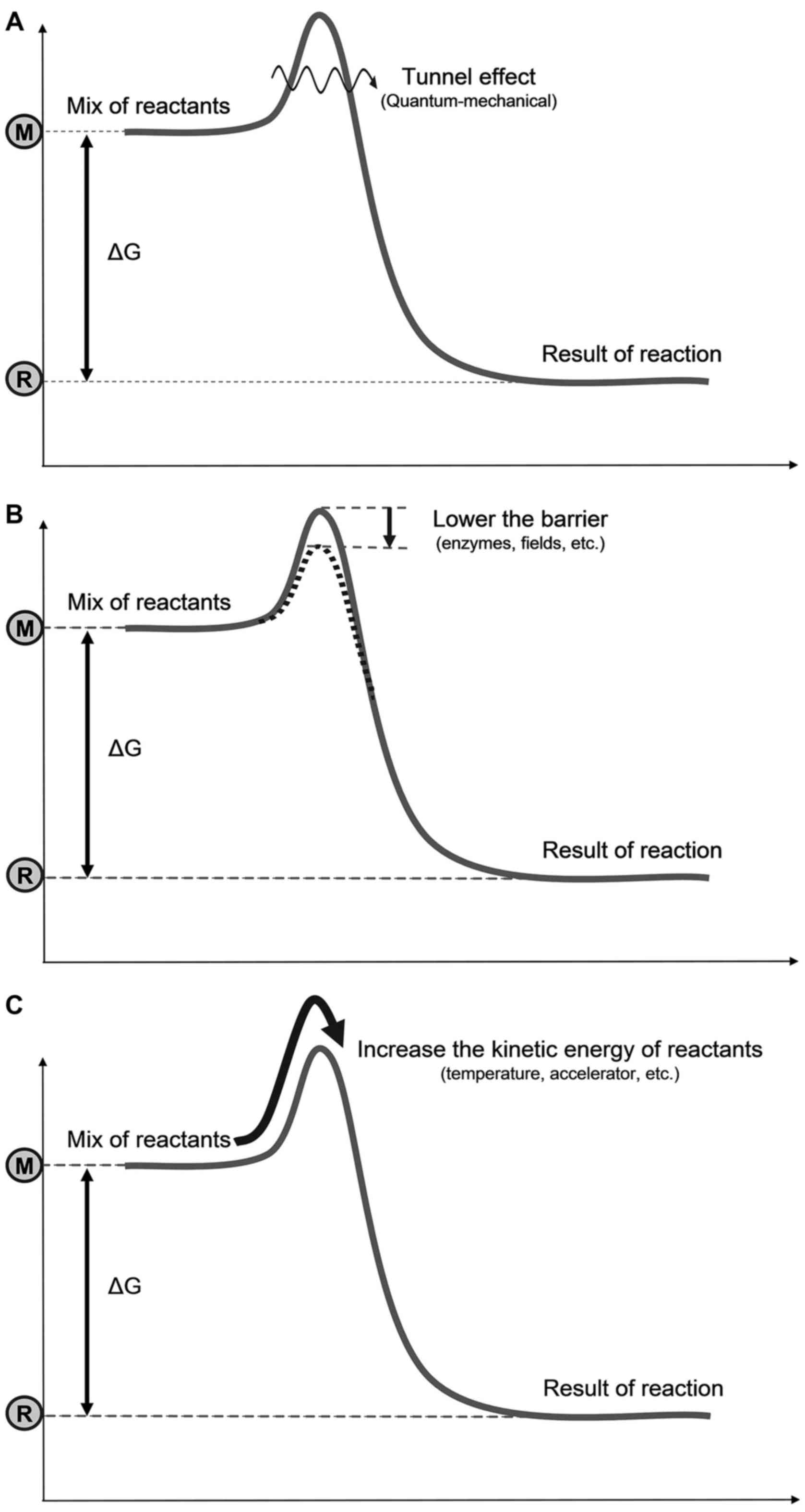
kr=Ae−EαRgT where T is the absolute temperature, (measured in K), Rg is the universal gas constant (Rg=8.314Jmol⋅K) and A is the ‘pre-exponential factor’ which defines the frequency of collisions, describes the irreversible jump, and measures its dimension in [1/sec]. The Arrhenius equation is an empirical observation, but is precise for most practical applications (11). A detailed history and trends have been described (12).Three possible mechanisms are available to go over the barrier (Fig. 3): i) Bypass the barrier: A quantum-mechanical (tunneling effect), non-temperature dependent solution (13); destroy or lower the barrier, or form an intermediate compound (transition state); Provide ease for going over the barrier; promoted chemically (e.g., enzymes), electromagnetically (e.g., an electric field), or by other effects; this is a non-temperature dependent solution; iii) surmount the barrier: i.e., A temperature dependent solution by increasing the kinetic energy of the reacting particles, by accelerating them individually or increasing their average kinetic energy (temperature). Note that two of these three mechanisms are not temperature-dependent, but are clearly energy consuming; thus, they are thermal.
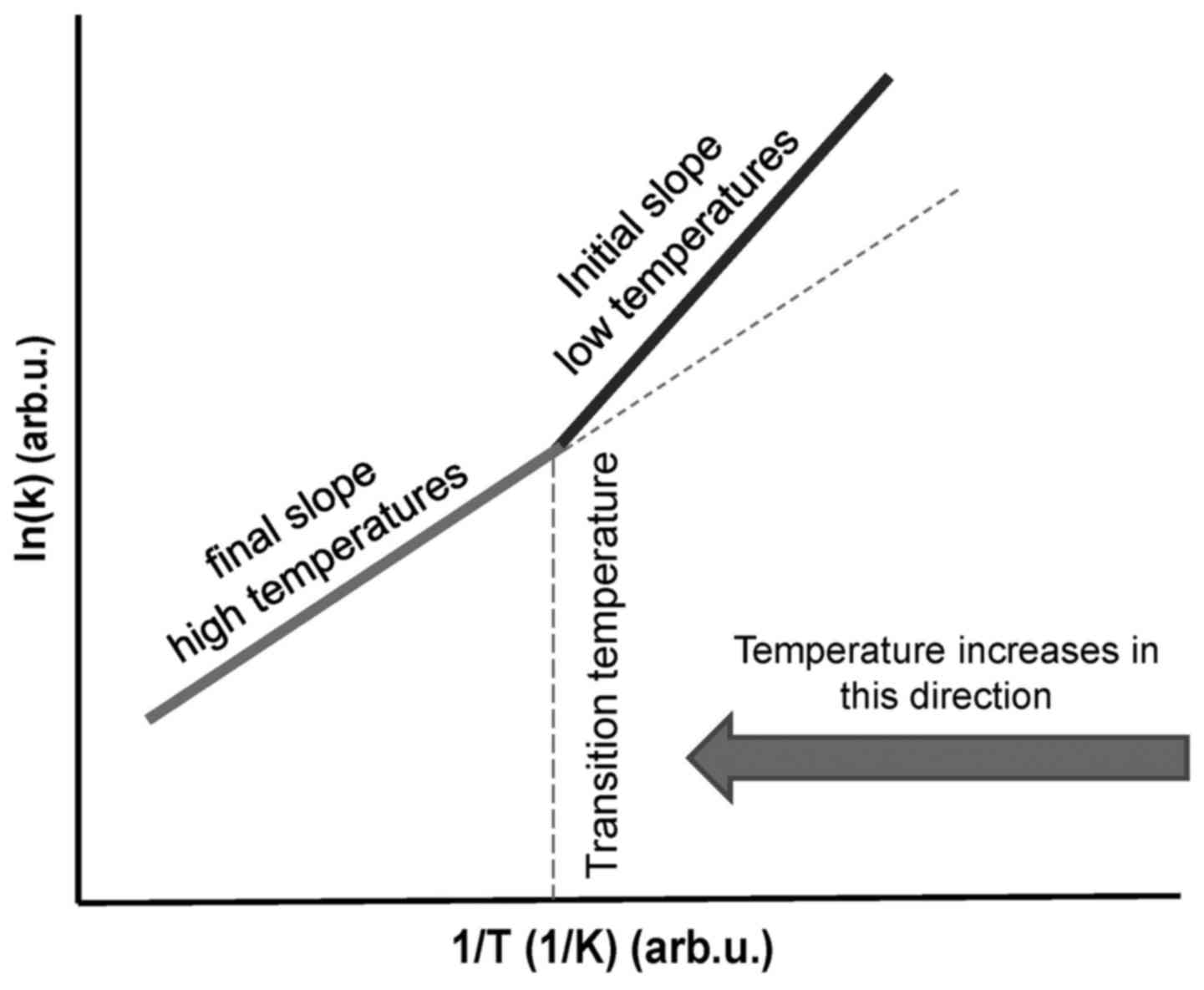
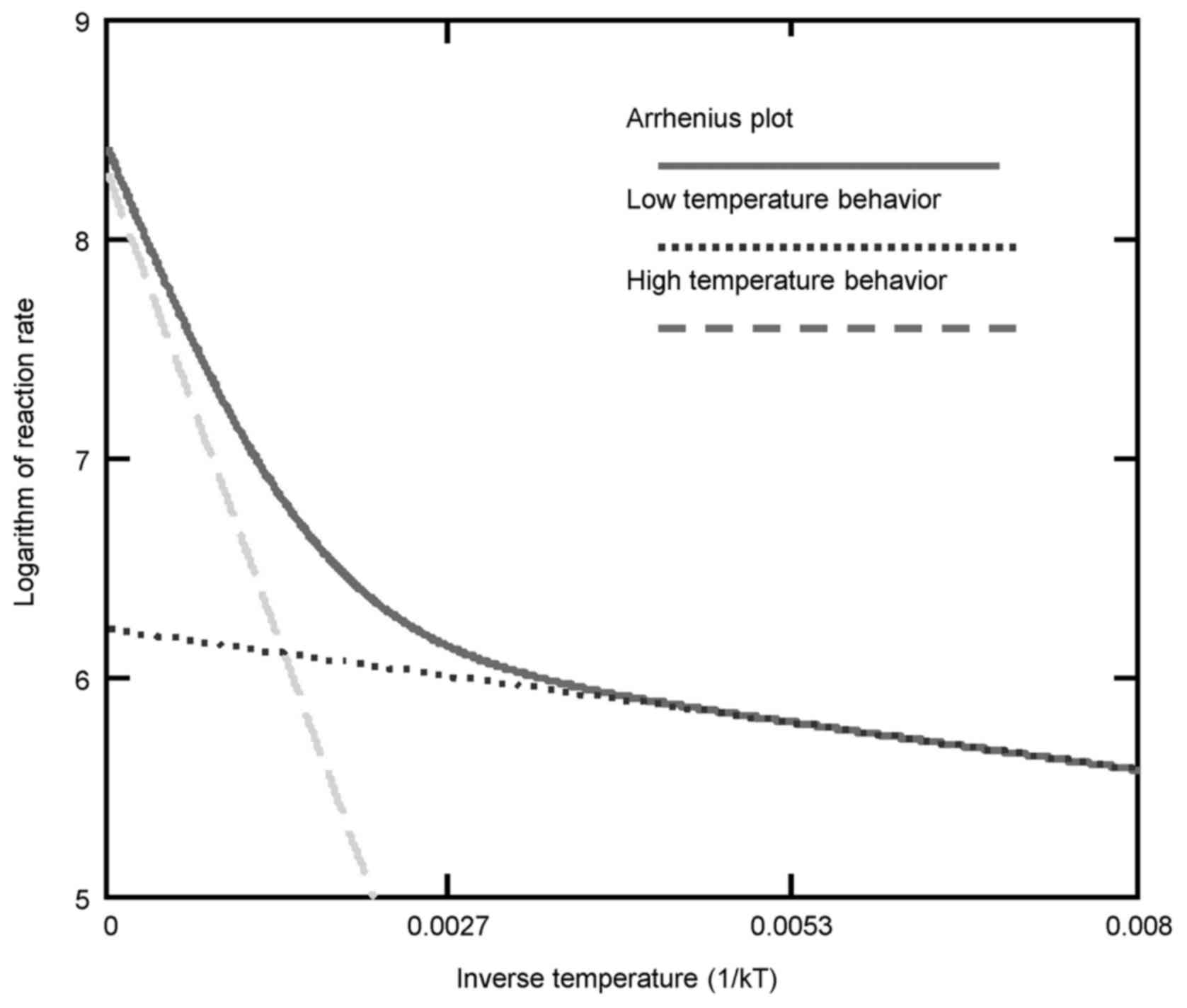
When a phase transition occurs, the activation energy (Ea) changes and a kink appears on the straight line (Fig. 4). The temperature of the kink is responsible for the transition, and the system remains at this point until the complete transformation has been performed. The kink in the Arrhenius plot is not fixed at a particular temperature, as it may be shifted by various heating processes and by chemotherapy (14) or other chemical conditions (15) that modify the actual reaction (16). An electric field also may affect the kink of the Arrhenius plot (17). The Arrhenius kink, which must be overheated, corresponds well to the believed cellular phase transition observed at around 42.5°C (18).
When the transition occurs non-homogeneously in the system, then the kink is not a point; it will appear as a curvature on the plot (19,20). The coexistence of two phases could occur for a wide range of temperatures, and then two (or more) Arrhenius lines combine to form the curve (Fig. 5).
When the reaction coordinates change, the system can be approached by the empirical Arrhenius functions. In this case, the process is definitely thermal. Sometimes categories of ‘non-thermal’ or ‘athermal’ effects are incorrectly used when the process is thermal but non-temperature-dependent.
The internal energy (U) of a system has a substantial variety of physical effects to be changed [Equation 2]:
where Yi and Xi are thermodynamic variables, intensive and extensive characters of the i-th component of the system, respectively. Possible intensive values could be pressure (p), temperature (T), chemical potential (μ), the electric field (E) or the magnetic field (H), while the extensive values (in order of their pairing with adequate intensives) are the volume (V), the entropy (S), the particle number (N), the polarization (P) and the magnetization (M). These thermodynamic values add energy terms to the internal energy in individual pairs, and thus the internal energy with some of the interactions is as follows [Equation 3]: ΔU=pΔV−TΔS+μΔN+EΔP+HΔMThis type of non-temperature dependent case occurs in transitions when the internal energy changes at a constant temperature.
Determining the correct the dose of hyperthermia
A well-defined and strictly scientific dose of hyperthermia therapy is a critical issue in research and is crucial to the future of hyperthermia in oncology (21). The proposed general definition of oncologic hyperthermia is forcing cell-damaging energy absorption on malignant cells and sensitizing other therapies. Accordingly, we have to control the damaging thermal effect on malignancy by an appropriate dosing process.
Consequently, the proper dose must be readily applicable to all technical solutions and reliably measured under standard hospital conditions. It must be measured quickly, with acceptable accuracy, and it has to be the basis of the comparison of different treatments by technical variants in clinical prac-tice. The proposed dose at present (22-24) is the cumulative equivalent minutes referring to 43°C: CEM43Tx (measured in minutes). It contains two parts. The CEM43 part is a time-dependent function FCEM43°C(V,t) [Equation 4]:
where V is the volume of the target (the energy is focused on the tumor mass; we assume that only the tumor volume is heated), t is the time and T(τ) is the time-dependent temperature, measured in °C. The R-value is fixed from the kink of the Arrhenius curve measured by in vitro experiments on Chinese hamster ovary cells (25).The FCEM43°C(V,t) function of Eq.4 is extended by a space-time dependent function Tx(V,t), where is the V is the volume of the tumor and x is given in % indicating the average percentage of monitored sensors (Vx portion of the tumor volume) in the V-volume where the temperature is on average Tx(V,t) [Equation 5]:
where Vx is the volume, where the temperature of the monitored sensors on average is Tx(V,t) and thus x=100Vx/V [%].Tx(V,t) is approximated mainly by guessing, and is usually guessed independently of time characterizing the percentage of the tumor which obtains the given minutes by CEM43°C. The missing parameter is real-time mapping, which could change the Tx(V,t) function in real-time, and would be necessary for a correct determination. Due to inhomogenous heat distribution, Tx decreases by growing monitored volume V. The dose is finally [Equation 6]: CEM43Tx=FCEM43°C(t)[min],condition:Tx(V,t){[°C],[%]} which is measured in minutes, but additionally considers the Tx(V,t) conditional function as a reference of in vitro necrosis at 43°C and two other physical units: A unit for the temperature (K or °C) and a unit for the portion of the isothermal average x (%). For example, CEM43T90 measures the cumulative equivalent minutes referring to necrotic cell killing at 43°C when the measured temperature is actually T90 in 90% of the monitored sites (referred to as the thermal isoeffect dose in 90% of the area) (23).This does use the time in non-conventional units (min instead of sec), and the unit is rigorously somehow a mixture of time and percentage. Non-linear physiological feedbacks need real-time measurements, which are also requested due to the integrative basis of the CEM43Tx dose. Furthermore, this dose value requires registering the temperature distribution of the target in space and time, which is practically not measurable.
Taken together, given the abovementioned challenges, the CEM43Tx dose has another severe scientific problem: The T(τ) has of course a dimension, °C. When we measure the temperature in other units (such as °F or °R), the value of CEM43Tx will be different if its unit does not contain the unit of the temperature. In the assumed mathematical form, R43-T(τ) has no dimension, which is not scientifically viable.
Moreover, CEM43Tx is controversial, and has failed to show the local control characterization of clinical results in soft tissue sarcomas (26), but was in line with clinical results for superfi-cial tumors (27). The administered dose of CEM43T90 for local hyperthermia did not exhibit an association between dose and clinical outcomes (such as local remissions, local disease-free survival and overall survival) (28). It has been calibrated by in vitro experiments (26), which are far from the reality of human medicine. Its necrotic reference at 43°C renders this dose unrealistic, as in most human hyperthermia treatments such a temperature is not reachable in the whole tumor. While the high temperature is realized in the ablation-like locality, the dosing by CEM43Tx was false (29). The inapplicability of this in vitro-calibrated dose is echoed in the whole-body hyperthermia (WBH) application, in which CEM43T100 is very high (T100 means the complete isothermal heating of the tumor), although the results are very different from the same dose provided by local hyperthermia of the tumor lesion (30).
Sometimes, the dose CEM43Tx is further cumulated by a number of treatments, i.e., 5 repeated treatments of 90 min each, having a different temperature definition, but using T90, which is the average temperature of 90% of the tumor volume (in the case of point sensors, 90% of the sensors) during the 60 min treatment time (31) [Equation 7]:
The CEM43Tx in general use depends on the number of sessions (N), the duration of the nth session (tn) and of couse Tx is also time-dependent [Equation 8]:
This cumulation needs to be assessed for the additive effects of the applied sessions independently of their repetition frequency. It is likely that a more extended period between sessions will have a different effect than frequent repetition, due to many changes in the level of stress proteins and the proliferation rate of the remaining living part of the tumor, although these are not considered.
The lack of a consensus on dose is the most substantial blockade to hyperthermia applications. A further challenge is that it is almost impossible to measure among standard conditions; it supposes measuring the temperature. However, the measurements must describe a map of the distribution, which needs many measured points in a tumor. This can be done invasively once. In most publications, the researcher measures the temperature intraluminally near the tumor. This indirect measurement of tumor temperature could skew the whole hyperthermia process. Usually, the intraluminal applications are not precise, as the physiological conditions (mainly the blood-flow) produce different temperature there than in the tumor. Equal energy absorption heats the probe in the lumen to a higher temperature. There is no guarantee that the lumen is heated at the same energy flux as the tumor. Introducing the specific absorption rate (SAR, W/kg) parameter over time (such as in radiation therapy, Gy=J/kg=SAR x time) would be a good dose option. Intensive surface cooling does not allow the correct energy dose; energy, which is taken away by cooling, is missing from the dose. The SAR and the temperature distribution are not identical (32).
There has been a weak attempt to modify the CEM43Tx and its cumulation by introducing the dose TRISE (33-35). TRISE is a custom-made thermal dose parameter based on T50 and the duration of heating, guessing the rise in the average temperature (Trise). TRISE emphasizes the rise in temperature concerning human body temperature. i.e., 37°C. This supposes that the size of the tumor does not change during the treatment, and the sensors remain in the same positions in the tumor. For example, when the treatment time is unified by every session (90 min), and 5 sessions are in the protocol with the same 〈T50n〉 (32), then [Equation 9]:
(using the original notations) [Equation 10]:Which is in a generalized form [Equation 11]:
More preciously [Equation 12]:
where ‘N’ is the overall number of sessions and ‘n’ is the successive number of treatments in the complete cycle of sessions, ‘tn’ is the duration of the actual nth session, and 〈T50(τ)〉 is the actual treatment average over Tx which is the temperature in x=50% of the monitored sites of the target. The average treatment, which covers the part of the target in general x is [Equation 13]:The Trise(N,tn) dose (measured in °C) is a definite temperature and differs entirely from CEM43Tx which is measured in minutes. The temperature is an intensive thermodynamic parameter, which means that it characterizes the thermal situation with an average independent of the mass or volume of the considered system. The temperature is, in the isothermal case, the same in every smaller part of the target, while we expect proportionality of the dose with the volume, i.e., half the volume (mass) would have half the dose. Here again, the possibility of adding subsequent treatments forming an overall dose is entirely missing.
Trise(N,tn) is approaching the SAR on 50% isothermal area of the tumor, and making great average on the inhomogeneities as well as on the time-variation of the absorbed energy. The correct dose (absorbed energy, AE) which transforms Eq.(12) scientifically correct and actually optimal [Equation 14]:
[14]Knowing that [Equation 15]:
In the adiabatic approach the average is [Equation 16]:
where ΔT is the temperature increase during the actual session. The Eq.(16) is similar, but more precise than Trise(N,tn) shown in [Equation 12]. The adiabatic conditions can be ensured by the well selected absorbing volumes and well controlled incident power.Systemic and local physiological effects introduce inaccuracies to the empirical doses in models in vivo, despite some actual measurements in preclinical research, such as a randomized canine trial show the significance of CEM43Tx dose (36). The complete dosing attempts by CEM43Tx and TRISE are in fact impractical and not applicable in daily practice, and their formulation is far removed from the criteria of scientific requirements for dosing.
The thermal effect
Generally, oncological hyperthermia is the result of energy absorption, which changes the target via chemical, structural, mechanical and electromagnetic variations (the induced anthropogenic fever therapies, or inflammatory local heating by biological effects are not discussed herein). Our topic is limited only for the energy-intake from outside (mostly electromagnetic) sources (37). These processes are constrained and the homeostatic control tries to re-establish the thermal equilibrium by physiological negative feedback effects, mainly by the regulation of vasodilatation. There are some other methods, such as fever production with drugs or biomaterials. However, the physiological mechanisms are opposite than the case by energy absorption; herein, the physiological feedback is positive, inducing the new type of homeostasis. Presently, we deal with only the energy-absorption based hyperthermic methods, which distort malignant cells. Energy absorption changes the temperature of the target in most cases. The temperature can be a tool for restructuring or merely signaling cell death and may orchestrate a complex set of molecules acting systemically against malignancy.
When all the absorbed energy is used for a phase change, the temperature will be fixed during this transition. Defining this effect as ‘non-thermal’ (38) is an innacuracy. A set of complex thermodynamic and bio-electrodynamic interactions is involved in the process (39), changing various physical characteristics, such as the chemical potential, the entropy, the dipole moments; or they may include in chemical reactions, polarizing molecules, producing an electric current, and so on.
Hyperthermia is a thermal effect on living subjects. Consequently, the Arrhenius function as the basis of the correct dosing (such as was selected for CEM43Tx too) is a perfect choice (22,40). The accurate fitting of experiments to the Arrhenius plot in vitro (41,42) is convincing regarding the thermal processes. The R-value changes according to the slopes of the Arrhenius plot. The kink in Arrhenius plot (43), with a sudden change of the slope of the line, is an excellent basis to fit the activation energies below and above the kink at a certain temperature, which provided the value of ‘R’ in [Equations 4 and 7]. However, the observed kink is an individual process probably connected to the phase transition. The temperature of the kink is reproducible under the same conditions and same cell line; however, it changes depending on the actual complementary chemotherapy (43,44) or actual cell or tissue (45). Due to the fundamentally complementary applications of hyperthermia, this change in the kink is frequent. The kink is not fixed under preheating conditions (46-49) and it is sensitive to the dynamism of heat transfer as well (50); thus, the R-value has to be adjusted to the actual position of the kink.
The kink in the Arrhenius plot is probably a lipid-associated phase transition (50-52), which could lower the activation energy to facilitate changes in the energy provided (53). The change in the kink is expected to be responsive to the blood flow as well (54).
When the kink happens in the Arrhenius plot at Tc
temperature, and the activation energies are Ea1 and Ea2 above and below it, respectively, then the generalization of R in the CEM-like formulation (55,56) is as follows [Equation 17]:
where T is the absolute temperature, measured in K. When T≅Tc, Rgen is constant. We may select Tc as the homeostatic body temperature, 310 K (37°C), as was selected in the TRISE proposal. This formulation efficiently introduces generalized cumulative equivalent minutes concerning Tc [Equation 18]:When the activation energies are Ea1 = 365 kcal/mol ≈ 1527 kJ/mol below this point, while above Ea2 = 148 kcal/mol ≈ 620 kJ/mol, below and above the kink point, respectively (18,41), then these values implicate Rgen(<43C)=0.159; Rgen(≥43C)=0.474, instead of the widely used R(<43C)=0.25; R(≥43C)=0.5, respectively. The error is below the robust breakpoint (~36%), indicating that non-necrotic damage cannot be described accurately with the standard CEM43°C dose. In in vitro experiments, x=100% in the Tx(V,t) function in [Equation 5]. This remarkable deviation occurs as the kink is artificial. The plot is a curvature in reality and depends on the spatial-temporal heterogeneity of the process. Thus, the extrapolated straight line is incorrect in the vicinity of the transition, e.g., the binding of adenosine triphosphate (ATP) to myosin subfragment-1 (46,51) or measuring the cytochrome c reductase activity of liver mitochondrial succinate for the heterothermic brown antechinus (57,58).
Making the CEM⎪Tc(t) value scientifically correct, independent of the measuring units of T temperature [Equation 19]:
Here, (Tc−T(t))Tc=ΔT(τ)Tcis free from the dimension (measuring unit) of the temperature, and Q=constant (when T≅Tc). Due to the high value of temperature in K, but its relatively small difference from Tc in this case [Equation 20]:The Arrhenius graph gives different time doses for the different points of the target (due to its non-homogeneous structure); this promotes chemical reactions and lowers the activation energy (59). In the case of multiple processes, the so-called ‘apparent activation energy’ Eaa should be introduced (60) [Equation 21]:
When the pre-exponential factor has a temperature independent and temperature dependent part, a constant ‘A’ can be separated from the temperature; thus, Eaa is the independent part of the temperature, and thus (61) [Equation 22]:
kr=ATme−EaaRgTIn practice, it is a rough linear slope fit on the measured ln(k) vs. (1/T) function, where a deviation from the slope will refer to differences in the actual chemical reactions. Of note, a complex chemical reaction, such as cooking can be described with such apparent activation energies (62).
The Arrhenius curve is most frequently measured in vitro, where necrotic cell death is considered to be the dominant thermal effect under cell culture conditions. In cases of pure thermal necrosis in vitro, the cell membrane is lethally damaged [e.g., due to protein denaturation (63)], and the damage point changes the kink of the Arrhenius plot depending on the kind of heating (64). Even the protein folding kinetics exhibit an Arrhenius temperature dependence when corrected for the temperature dependence of protein stability (65) and = trans-membrane protein-lipid and protein-protein interactions (66).
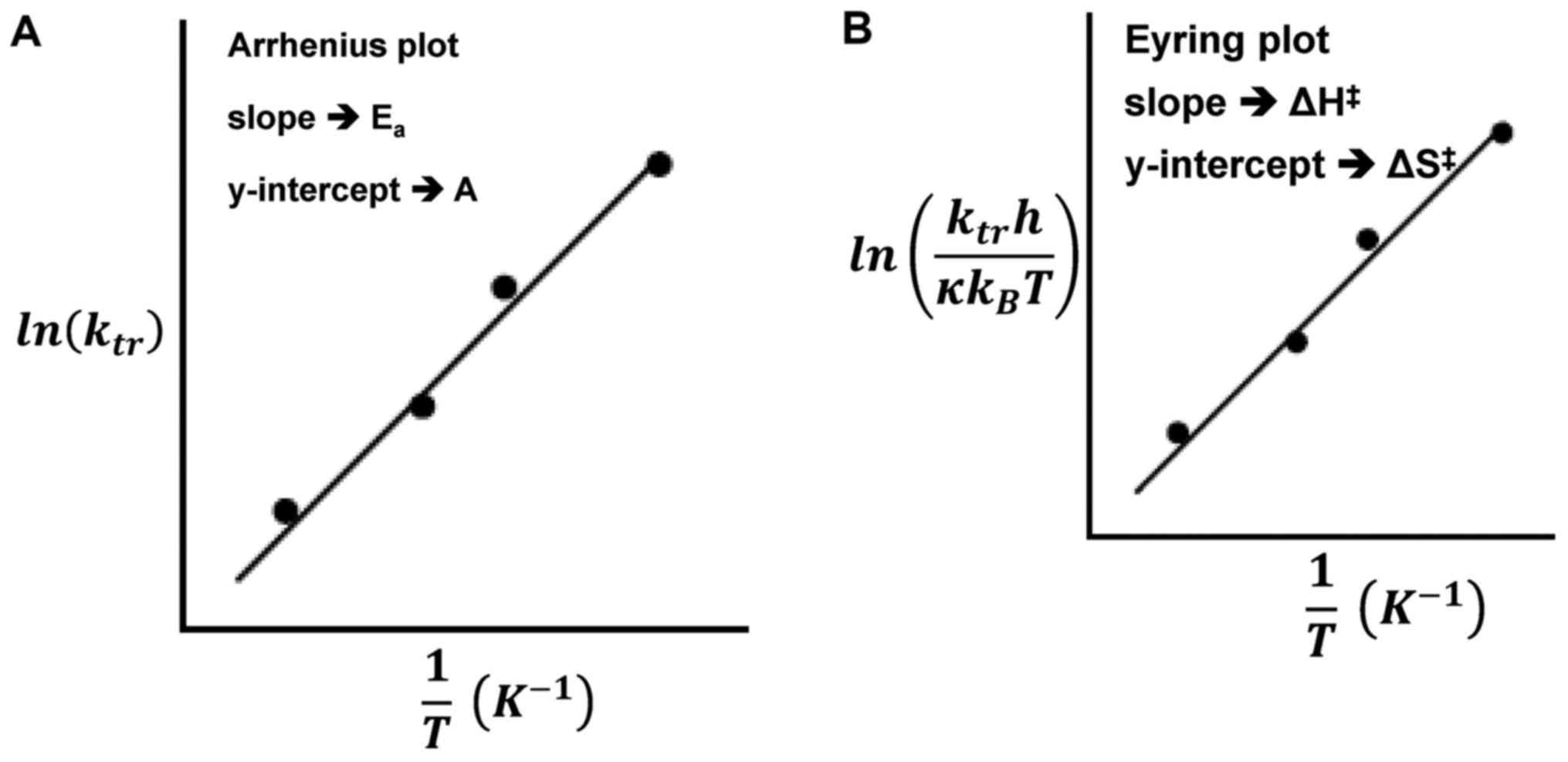
The Arrhenius mechanism has a more sophisticated theory, using the variance in the reaction rate by temperature to study the transition phase of the reaction on the energy of the barrier (67,68). In this manner, the semi-empirical Arrhenius equation was rigorously derived from statistical thermodynamics (69), using the enthalpy of activation together with the entropy change in the same process. The transition state of this complex process has been described (20) and further developed (70) for cell death, which approaches practical applications for the thermodynamic description of the multistep complex biological progression of interactions. This rigorous thermodynamic explanation has generalized the thermal dose with improving accuracy possible (71). Eyring and Polanyi (Eyring et al, Wynne-Jones and Eyring H, Eyring and Polanyi, Eyring, and Eyring and Stearn) developed a theoretically well-supported modification of the Arrhenius plot (68,72-75), which was developed further in Fig. 6 and can be used in quantum-mechanical chemistry (76).
The vital assumption of the transition-state theory is that the transition state is in equilibrium with the reactants and products in the state of the activated complex (11).
The Eyring formulation reforms the reaction coordinates of the conventional empirical Arrhenius plot. The final product develops steadily from the reactant configuration through a transition state. This interim phase is a cluster configuration of the activated transitional complex. In this approach, the reaction coordinate follows the gradient path of potential energy from the initial reactants to the final products, e.g., in simple reactions, the lengths of bonds can be chosen as the reaction coordinate. The transition state population [Equation 23]:
where ΔG# is the free energy of activation (transition state), kB is the Boltzmann's and h is Planck's constant, υ is the vibration frequency of activated complex and ktr# is the population of the transition state, and the constants are as follows [Equation 24]: kB=Rg6.022·10−23m2kgs2K≅1.38·10−23,h≅6.626·10−23m2kgsNote that the factor (kBThv)is the ratio of the actual thermal and quantum-mechanical energy.
From these, Eyring's expression is formally similar to that of Arrhenius [Equation 25]:
Where ‘κ’ is the transmission coefficient; it is the probability of oscillation of the activated complex in the transition state leading to a product. The difference, however, is essential: While the Arrhenius plot describes an experimental macroscopic rate constant for the entire transformation process, the Eyring plot deals with microscopic transitions as fundamental steps.
Due to the thermodynamic conditions [Equation 26]:
ΔG#=ΔH#−TΔGS# where ΔS# and ΔH# are the entropy and enthalpy of activation. For simplicity, we use the molar values of the extensive thermodynamic parameters [Equation 27]:Using [Equations 25 and 26], we get the following [Equation 28]:
A comparison of [Equations 28 with 1] makes the pre-exponential frequency factor and the activation energy in empirical Arrhenius function scientifically accurate [Equation 29]:
This result provides an essential explanation for the theoretical background of the pre-exponential factor and the activation energy. The ΔH‡ enthalpy is the kind of energy manifested in a transition state, while the ΔS‡ entropy represents the energy ‘trapped’ during the interactions.
The Eyring and Arrhenius plots are similar to the fitting of the curves does not differ in the physiological range (77); however, the slope and intercepts have different meanings in Fig. 7. The significant difference is that while the ordinate shows the logarithm of the reaction rate ‘k’ in the Arrhenius plot, in the Eyring plot, the ordinate has a temperature dependent addition. The superiority of the Eyring model is its theoretical background compared to the empirical Arrhenius model. The central fact that we can use in hyperthermia is that the pre-exponential factor of the Arrhenius plot is entropy dependent. The entropy abruptly jumps during phase transitions, with immediate changes to the chemical structure, which is the essential requirement of hyperthermia.
The Arrhenius parameters (Ea and A) are functionally connected empirically (78) [Equation 30]:
Eα≅2.61⋅103ln(A)+2.62⋅104Or [Equation 31]:
ln(A)≅3.832⋅10−4Eα−10.042 while others (79,80) have slightly different values [Equation 32]: Eα≅2.63⋅103ln(A)+2.46⋅104Or [Equation 33]:
ln(A)≅3.8⋅10−4Eα−9.36The linear plots of the Arrhenius parameters [Equations 30, 31, 32, 33) are arithmetically given the same linear dependence of the molar entropy and molar enthalpy due to the Eyring connections, such as seen below:
If, due to [Equation 29] the below are obtained [Equation 34]:
Eα=H‡≅cln(A)+d(c and d are constants) and [Equation 35]:
where q is constant. In consequence [Equation 36]: where a and b are constants.It is indeed observed. A collection of data on molar entropies and enthalpies are found in numerous irreversible thermal denaturalization references; a linear dependence can be seen between the change in molar values (81).
Hence, in case of the processes collected in, the following are obtained [Equation 37]:
ΔS=αΔH+b where b=-327.5 [J/mol/K] and a=0.003147 [1/K]; others (82) measure b′=-271.7 [J/mol/K]; a′=0.0030395 [1/K] according to the best fit of the line.Using the thermodynamical equivalence in [Equation 26] the following are obtained [Equation 38]:
This expression offers the ability to control the accuracy [Equation 39]:
and [Equation 40]:The above-calculated temperatures fit the experimental conditions. From this, the change in the free energy is as follows [Equation 41]:
ΔG≅104,067[J/mol] and [Equation 42]: ΔG′≅89,389[J/mol] which is in the range of the usual experimental expectations.The main consequence of this observation is a clear picture of robustly used energy utilization for chemical changes and differs from the process which leads to an increase in the temperature only.
These processes probably represent different molecular reactions, which require further investigations. This shows that the absorbed energy is utilized for different purposes, not only for raising the temperature. When we intend to change the structure, all the energy components (the chemical reactions, the mechanical properties, and so on) require energy, and the rest will be distributed equally to increase the average kinetic energy of the part of the system. Consequently, the energy that is not used for particular purposes will ‘invested into the pool’ and will increase the temperature. The increasing temperature represents energy that has no specialized tasks, and thus in this regard, it is wasted energy.
Furthermore, we know another linear expression of molar entropy for the temperature adaptation of enzymes (83) [Equation 43]:
where and K (sec−1) = (Vmax/mg of enzyme) × (molecular weight) × (10−3 mmol/µmol) × (1 min/60 sec), where the molecular weight of the enzyme is expressed in mg/mmol.The connections between the activation parameters are observed in cases of muscle-type lactate dehydrogenase, D-glyceraldehyde-3phosphate-dehydrogenase, and muscle glycogen phosphorylase b.
To verify the effect of hyperthermia, thermal damage is measured in multiple experiments. CEM usually character-izes the damage dependent fit to the Arrhenius plot and accurately fits the Arrhenius plot in vitro (41,43). According to [Equation 7], the proportional fitting value R depends on the rate of thermal destruction. Since this destruction rate varies according to tissue type, R is not constant at all (84).
Concept: Dose model of modulated electro-hyperthermia
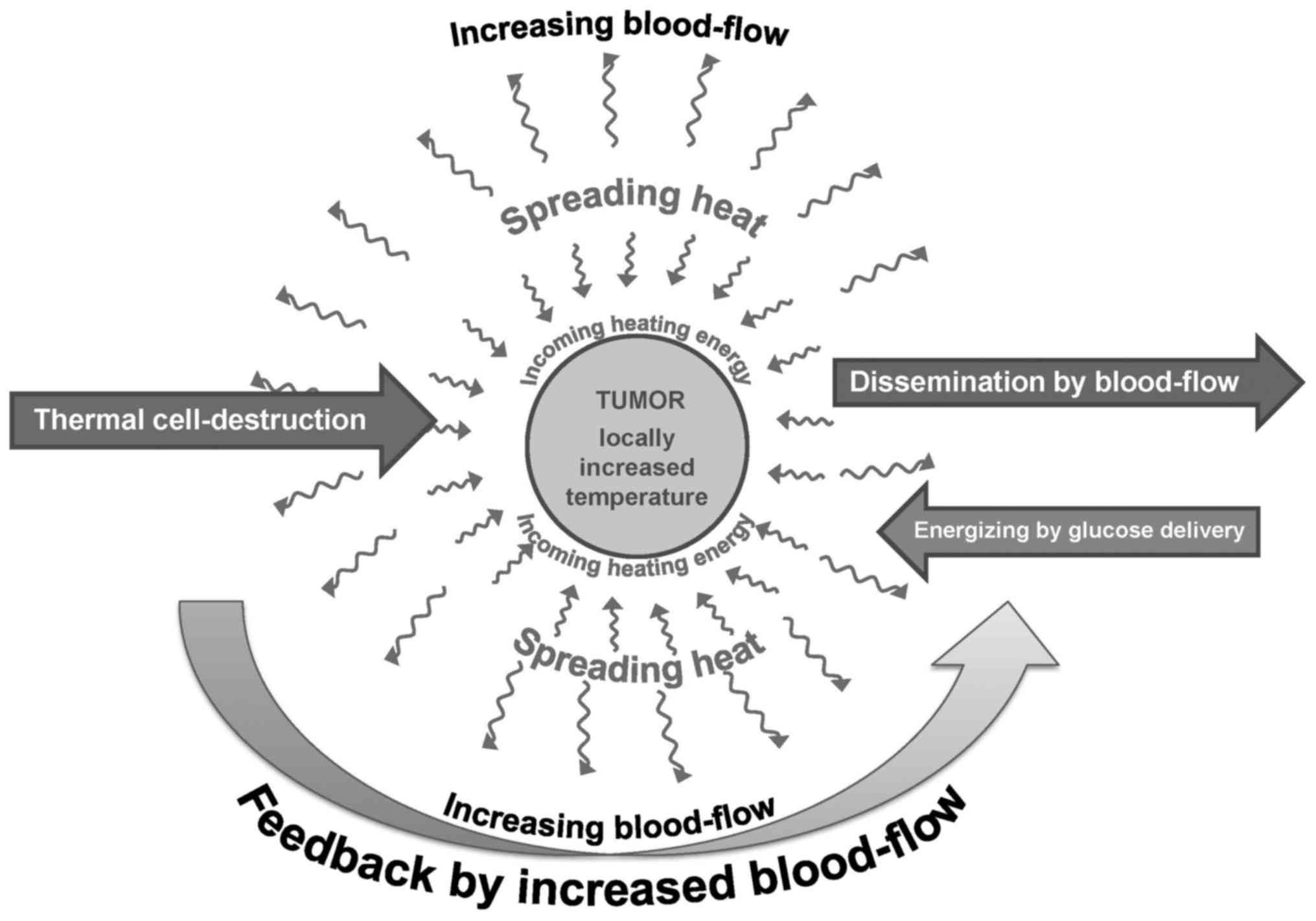
The variability of destruction, as well as the challenge of the previously described competitive effects of thermal damage and blood flow, together with intensified dissemination, need another concept than the pure thermal destruction demonstrated in vitro. The thermal process has to be sufficient for the disruption of malignant cells and for sensitizing complementary therapies; however, along with these effects it must not increase the blood flow to a high degree, as this has the deleterious effects of increasing the tumor's nutrient supply and the dissemination of malignant cells, as shown in Fig. 8.
A new type of hyperthermia appears to have solved this challenge: Modulated electro-hyperthermia (mEHT, trade name oncothermia) (79). This method is devoted to using non-temperature dependent thermal effects, which are capable of triggering apoptotic processes by specific molecular processes. We disapprove of the concept of the isothermal heating of the whole tumor mass, avoid the controversial effect which is caused by the feedback of thermal homeostasis, which uses the non-linear control of blood-flow. The energy absorption by the individual malignant cells uses the energy where it is necessary, to initiate apoptotic signal transductions. In consequence, the measured temperature and the stimulated blood-flow are mild (85), but the local control and the overall survival are both increased (86). In this manner, mEHT leads to selective heterogenic heating, genuinely breaking the homogenous, isothermal concept.
The mEHT method uses the biophysical differences between healthy and malignant cells for selection of its action, precisely heating in a nanoscopic range and igniting molecular reactions (87). The selection exploits the character of malignant cells, which indeed differ from their healthy counterparts. Healthy cells are in a communicative network, maintaining the complexity of a well-controlled system, while malignant cells are mostly autonomic, having lost their ‘social’ connections with other cells, and fighting for with every other cell nutrients, irrespective of their health status. The existence of the network categorizes cells as connected or non-connected (88). Malignant transformation can be regarded as a phase transformation (89), as cells lose their multicellular behavior (90) transforming the highly complex network into a set of individual cells (91,92). This process is similar to reverse evolution (atavism) (93). However, it is different from prokaryotes. These cells are well-developed eukaryotes with complete genetic content, although they have lost their collective complexity, and their mitochondria are not able to supply the enormous energy demand for permanent proliferation.
The consequences of malignant differences indeed provide the possibility to distinguish them from healthy cell arrangements (94). This differentiation is based on the contents of the extracellular aqueous electrolyte. The higher metabolism of malignant cells needs a robust amount of glucose for ATP production, which is measurable by positron emission tomography (95). In cancer cells, ATP production is predominantly performed by simple anaerobic glycolysis instead of mitochondrial phosphorylation. This rapid, intense process produces lactate which, together with the higher transport of other ionic species, considerably increases the ionic conductivity of the electrolyte in the extracellular matrix of the tumor. An applied radiofrequency (RF) current prefers flowing through the low resistance tumor than the healthy environment. This effect is measurable by RF current density imaging (96). The RF current predominantly flows in the extracellular electrolyte. Its energy-absorption creates an active temperature gradient through the membrane (97).
This tumor selection is accompanied by a cellular difference, making the selection microscopic. In the mEHT method, the overall physiologic feedback loops are not promoted by massive general temperature increase of the targeted volume; mEHT functions as mild hyperthermia macroscopically, while it functions as extreme hyperthermia microscopically for malignant cells. Malignant cells are autonomic and break their intercellular communications, i.e., contacts via adherent proteins and junctions mostly vanish. This difference in the structure of the extracellular environment in the near vicinity of cells (98) renders them distinguishable by their dielectric permeability (99,100), for which the applied RF current flow is discriminatory. This is a well-developed diagnostic method (101) that is applied in mammography (102).
Furthermore, the complete pattern of the malignant tissue differs from the pattern of healthy tissue, which is used for pathological image recognition in biopsy samples. The pathological pattern modifies the spatiotemporal interactions of the cells, which is not a static pattern but dynamically acts via intercellular interactions. These dynamic relations produce a noise of homeostatic equilibrium, which is measured as a peculiar signal (103,104). This noise differs in malignancy versus healthy tissue (104) and is measurable by the RF current (105). The noise difference is the basis for the applied modulation on the RF carrier. The modulation is one of the important features of mEHT (oncothermia) method (106). The modulation is an information delivery to the malignant lesion. The applied time-fractal has such autocorrelation time-lags that well fits to the apoptotic excitation processes and also may reconstruct the broken E-cadherin-beta-catenin cellular connections (107), in repeated independent measurement as well (108).
Technically mEHT couples the electromagnetic energy by high-precision impedance matching (patented), which renders such tight coupling similar to it galvanic. Reflection at 150 W incident is <1% (1 W) in the maximal output of oncothermia device. The conventional hyperthermia devices use 10 times more power than mEHT; however, most of that vanishes by various energy losses (radiation to the air, high energy reflection, heating the electronic parts, etc.). Consequently, they must measure the temperature in the target having idea about the absorbed energy there. The innovative tight coupling allows to use the energy as dose of the mEHT treatment (85).
The above-mentioned selection steps guide energy delivery; using β-dispersion involving bound water on the membrane (109,110). The broad range of β-dispersion has a part denoted by δ, (111), which is primarily selective for transmembrane proteins. This effect indices real energy absorption, which happens at clusters of transmembrane proteins (112-114). All these publications above show the excellent selectivity of the mEHT method, acting on the membrane rafts of the malignant cells, while do not harm any healthy cells, having properties which are well distinguishable by the above-described biophysical factors from the malignant cells (87). The mEHT makes the same apoptosis as conventional hyperthermia does but in lower temperatures by 3°C (115,116), which is the consequence of the absorption of the energy in transmembrane proteins in the rafts.
Selection and energy absorption by a modulated RF current induce cell destruction beyond simple thermal effects. The in vitro research shows the absolute differences of mEHT from the conventional heating like water-bath or simple capacitive coupling (108,115). The in vitro proven increased efficacy has been supported by in vivo research (117) and continues to prove the feasibility in preclinical (118) and clinical applications (119) as well.
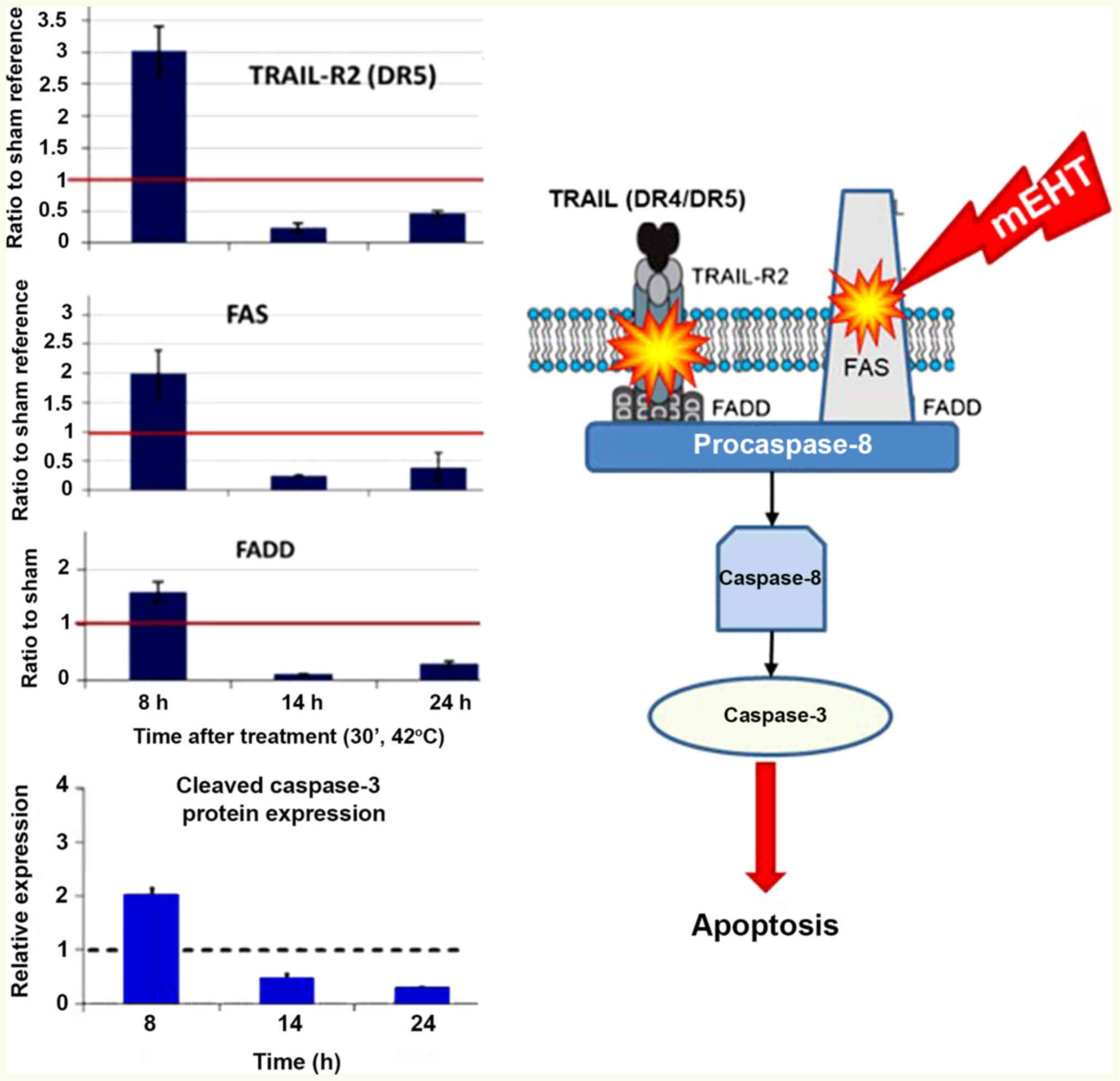
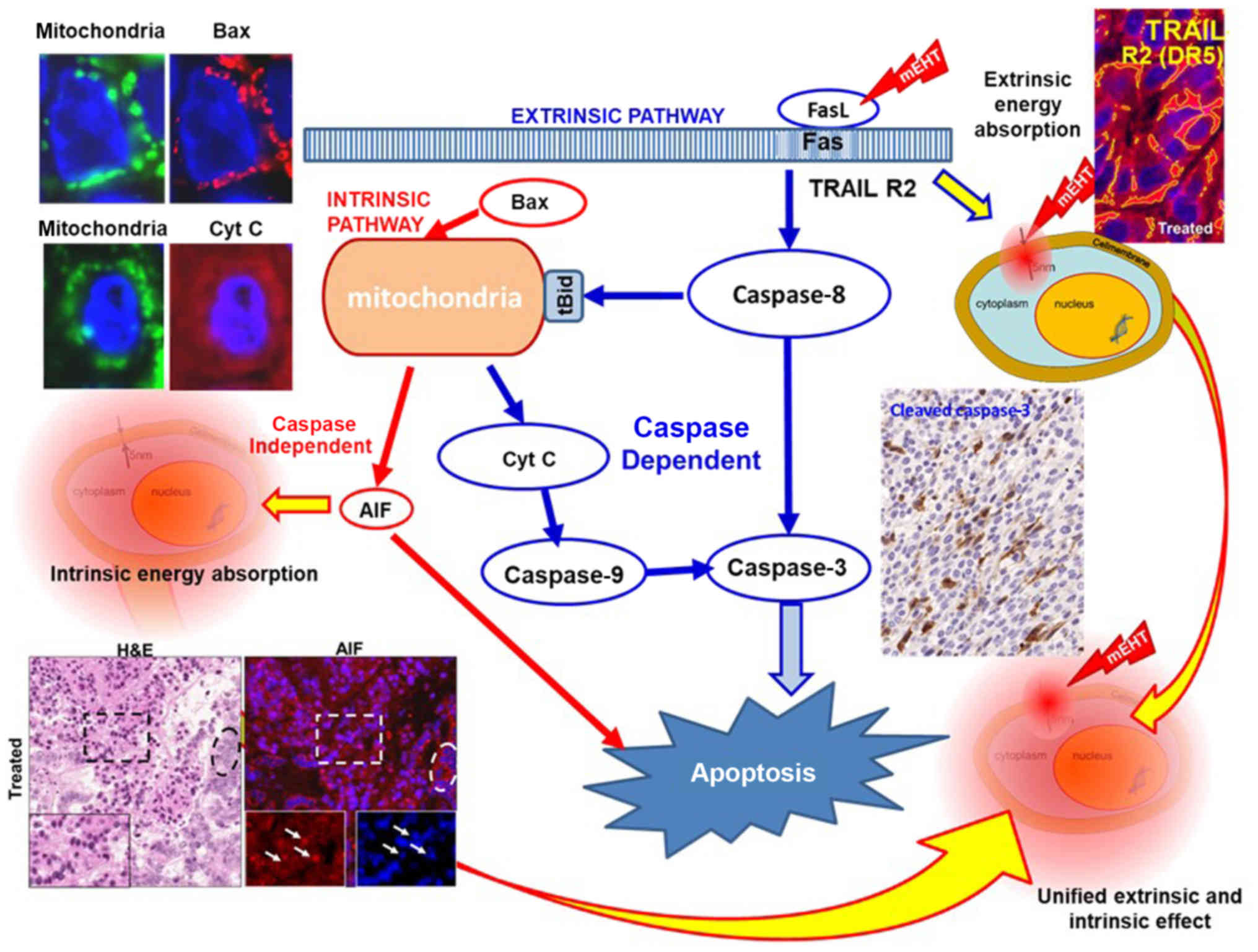
The absorbed energy induces a particular apoptotic process in transmembrane proteins (113), which energy is absorbed by the membrane rafts (structure of transmembrane proteins). This energy promotes the extrinsic pathway of apoptosis through the TRAIL-FAS-FADD complex, leading to cleavage of executor caspase-3 and induction of the apoptotic process, as shown in Fig. 9 (120). Additionally, the activation of caspase-8 could trigger intrinsic pathways together with the cell-wide spread of heating energy. These intrinsic pathways include caspase-independent [activate apoptosis-inducing factor (AIF) (121)] and caspase-dependent apoptosis through cytochrome c (122). Furthermore, the pro-apoptotic cell death-related gene network (such as EGR1, JUN and CDKN1A) in induced, as well as the cryoprotective gene network (HSPs) (116). Various pathways are united in the final mechanism of action (Fig. 10).
The destruction of the malignant cell is dominantly apoptotic by the above signal excitations (120) developing a damage-associated molecular pattern (DAMP) (121), is the basis of immunogenic cell-death (ICD) (122). These processes prepare antigen recognition cells to produce helper and killer T-cells with direct recognition of the malignant cells over the body, finding the disseminated cells and distant metastases, (abscopal effect) (123,124).
This targeting of the extrinsic pathway requires much less energy than heating the complete mass of the tumor, and furthermore, it is a direct apoptosis induction, which is not the case in the isothermal heating. The properly selected energy absorption is the reason why mEHT uses considerably less energy than conventional hyperthermia (125), while its results are showing significant improvement of both the local control and survival time (86).
Thermally activated non-necrotic cell-killing has a point of no return, where the process becomes irreversible, and the cell is committed to die. One of the pathways is BAX-mediated pore formation on mitochondria and the release of cytochrome c. Apoptosis and necrotic cell death are complex processes involving numerous chemical reactions and required a long period of time to be completed (126). The apoptotic signals begin the process, which is at the beginning, reversible. There are some points (depending on the signaling pathway) which are the ‘points of no return’ from where the process automatically continues and finishes by positive feedback constraint. In principle, the final phagocytosis of apoptotic bodies could be 48 h after the process has commenced.
One of the characteristic points of no return is the release of cytochrome c from the mitochondria (127), which usually takes 4 h after the initial apoptotic signal. Apparent activation energies associated with protein dynamics show relatively simple straight-line Arrhenius plots, so deviations from the single reaction plot are acceptable (128). In most reactions in living systems, Arrhenius plots are linear (no kink) below 42°C, e.g., in the mitochondrial membrane (54,129) and BAX-mediated pore formation (130,131) which is connected to cytochrome c (132,133), the appearance of which is one of the points of no return. Essential variations in cell polarity (134), enzymatic reactions (135), signaling pathways (136), or the activation of the hsp27/p38MAPK stress pathway (137) could help destroy or modify cancerous tissues.
A single Eaa can describe these processes as the kink of the Arrhenius plot is outside the investigated temperature interval. Using the Eaa apparent activation energy in complex reaction-kinetic processes of apoptosis can be formally converted to the apparent molar enthalpy and molar entropy. The entropy shows the energy part, which is trapped in the chemical and structural changes caused by the treatment. For dosing, we have to choose a parameter characterizing the process of non-necrotic cell killing.
The Eyring (Arrhenius experiment) plots show the possibility of the CEM-like behavior of the dose, as indicated earlier (20,55). However, the difference from the flawed CEM43°CTx is remarkable. No kink is considered in any of the curves of the various temperature dependences of various protein changes, only the slope (molar enthalpy) and molar entropy involved in the pre-exponential factor are used as proposed (20,55). This has specific disadvantages; however, we have to be sure that only apoptosis is characterized, which involves the actually chosen protein and indicates a definite commitment to apoptosis. This protein could be cytochrome c, cleaved caspase-3 or AIF. The signal transfer to apoptosis can occur through various ways.
The signal transduction pathways of apoptosis are mixed and produce a defined result. Thus, we have to use the same concept as that used for ionizing radiation. There, we know that the dominant effect breaks the strands of DNA, which anyway follows Arrhenius plot (138). Heat may also break DNA as shown by its Arrhenius behavior (139). With ionizing radiation, no calculations are made regarding the kind of damage (how many and which strands are damaged); the only point is the measurable result, which is proportional to the applied energy. By targeting breaks in DNA, isodose energy is used, assumed that all malignant cells have the same power to destroy their DNA strands. All the other absorbed energy, which makes heat or destroys parts of healthy cells, is regarded as a side-effect. The isodose condition, of course, has many side-effects, as malignant cells are only slightly selected under isodose conditions. The mEHT method enables the more precise selection since the target has self-selection mechanisms orienting the energy to the places where it acts optimally, which is measurable (113,120-122,140). The efficacy of energy delivery also must be optimal, which has been shown for mEHT (125). The mEHT method is highly personalized (55,141) and thus minimizes adverse effects. Consequently, the dose of mEHT could be the same as for ionizing radiation, with the energy defined as J/kg (Gy) or Ws/kg.
The above considerations are valid for single shot treatments. However, this is not sufficient, as clinical applications always use multiple sequential treatments. A series of sessions is applied clinically, so ‘fractional heating’ can be dosed. This requires clarification regarding the equilibrium of permanent cell death and the fresh round of cell proliferation, as well as the possibility of reverse chemical reactions. This problem overshadows fractional radiotherapy applications (142,143), as the repair of damaged DNA modifies the results of fractional therapy (144). In our fractional treatment method, this problem is less critical as apoptosis induced via the intrinsic and extrinsic ways is complete, no DNA repair is possible, and only the further proliferation of malignant cells leads to regrowth of the tumorous lesion. The critical result is that proliferation is suppressed in cells not destroyed in the target, measured by the Ki67 proliferation index (145).
Taken together with the molecular changes, the treatment capable of sensitizing the tumor to radio- (146) and chemo- (147) therapies, solving the challenge of the significant and controversial vasodilation induced by the conventional hyperthermia.
In conclusion, the appropriate dose of oncological hyper-thermia has to be the well-known energy-based concept, Gy (=J/kg). This dose is appropriate for the complex non-ionizing radiation process and also unites the complete radiation field (together with ionizing radiation) around the same dose control.
Funding
AMS is grateful for the support of the Hungarian Competitiveness and Excellence Program grant (NVKP_16-1-2016-0042).
Availability of data and materials
Not applicable.
Authors' contributions
SYL, GPS and AMS were involved in the conception and design of the study and worked together on drafting, revising and finalizing it. All authors have read and approved the final manuscript.
Ethics approval and consent to participate
Not applicable.
Patient consent for publication
Not applicable.
Competing interest
The authors declare that they have no competing interests.
Acknowledgments
Not applicable.
References
|
Nielsen OS, Horsman M and Overgaard J: A future for hyper-thermia in cancer treatment. Eur J Cancer. 37:1587–1589. 2001. View Article : Google Scholar : PubMed/NCBI | |
|
van der Zee J: Heating the patient: A promising approach. Ann Oncol. 13:1173–1184. 2002. View Article : Google Scholar : PubMed/NCBI | |
|
Roussakow S: The History Of Hyperthermia Rise And Decline Hindawi Publishing Corporation. Conference Papers in Medicine 2013; pp. 34280272013; | |
|
Oncology Encyclopedia: 2008, MedicineNet Hyperthermia definition, Answers. http://www.answers.com/topic/hyper-thermia. Accessed May 23, 2018. | |
|
Medicine.net: 2008, Hyperthermia definition. http://www.medterms.com/script/main/art.asp?articlekey=3848. Accessed May 23, 2018. | |
|
National Cancer Institute Hyperthermia definition. http://www.cancer.gov/about-cancer/treatment/types/surgery/hyperthermia-fact-sheet. Accessed May 23, 2018. | |
|
Wikipedia: Hyperthermia definition. https://en.wikipedia.org/wiki/Hyperthermia_therapy. Accessed May 23, 2018. | |
|
Medical Dictionary Hyperthermia definition. http://medical-dictionary.thefreedictionary.com/hyperthermia. Accessed May 23, 2018. | |
|
The Americal Cancer Society: Hyperthermia definition. http://www.cancer.org/treatment/treatmentsandsideeffects/treat-menttypes/hyperthermia. Accessed May 23, 2018. | |
|
Arrhenius S: On the reaction rate of the inversion of non-refined sugar upon souring. Z Phys Chem. 4:226–248. 1889. | |
|
Peleg M, Normand MD and Corradini MG: The Arrhenius equation revisited. Crit Rev Food Sci Nutr. 52:830–851. 2012. View Article : Google Scholar : PubMed/NCBI | |
|
Pollak E and Talkner P: Reaction rate theory: What it was, where is it today, and where is it going. Chaos. 15:0261162005. View Article : Google Scholar | |
|
Thomson WH: Quantum mechanical transition state theory and tunneling corrections. J Chem Phys. 110:4221–4231. 1999. View Article : Google Scholar | |
|
Urano M: Thermochemotherapy: From in vitro and in vivo experiments to potential clinical application. Hyperthermia and Oncology. Urano M and Douple E: VSP Utrecht; Tokyo: pp. 169–204. 1994 | |
|
Lin R, Chang DC and Lee YK: Study of temperature effect on single-cell fluid-phase endocytosis using micro cell chips and thermoelectric devices. 14th International Conference on Miniaturized Systems for Chemistry and Life Sciences; 3-7 October 2010; Groningen. pp. 962–965. 2010 | |
|
Rosemeyer H, Körnig E and Seela F: Dextran-linked purine nucleosides as substrates and inhibitors of adenosine deaminase. Eur J Biochem. 127:185–191. 1982. View Article : Google Scholar : PubMed/NCBI | |
|
Antov Y, Barbul A, Mantsur H and Korenstein R: Electroendocytosis: Exposure of cells to pulsed low electric fields enhances adsorption and uptake o. macromolecules = Biophys J. 88:2206–2223. 2005. | |
|
Dewey WC, Hopwood LE, Sapareto SA and Gerweck LE: Cellular responses to combinations of hyperthermia and radiation. Radiology. 123:463–474. 1977. View Article : Google Scholar : PubMed/NCBI | |
|
O'Neill DP, Peng T, Stiegler P, Mayrhauser U, Koestenbauer S, Tscheliessnigg K and Payne SJ: A three-state mathematical model of hyperthermic cell death. Ann Biomed Eng. 39:570–579. 2011. View Article : Google Scholar | |
|
Szasz A and Vincze G: Dose concept of oncological hyper-thermia: Heat-equation considering the cell destruction. J Cancer Res Ther. 2:171–181. 2006. View Article : Google Scholar | |
|
Jones E, Thrall D, Dewhirst MW and Vujaskovic Z: Prospective thermal dosimetry: The key to hyperthermia's future. Int J Hyperthermia. 22:247–253. 2006. View Article : Google Scholar : PubMed/NCBI | |
|
Dewhirst MW, Viglianti BL, Lora-Michiels M, Hanson M and Hoopes PJ: Basic principles of thermal dosimetry and thermal thresholds for tissue damage from hyperthermia. Int J Hyperthermia. 19:267–294. 2003. View Article : Google Scholar : PubMed/NCBI | |
|
Dewey WC: Arrhenius relationships from the molecule and cell to the clinic. Int J Hyperthermia. 10:457–483. 1994. View Article : Google Scholar : PubMed/NCBI | |
|
Perez CA and Sapareto SA: Thermal dose expression in clinical hyperthermia and correlation with tumor response/control. Cancer Res. 44(Suppl 10): 4818s–4825s. 1984.PubMed/NCBI | |
|
Sapareto SA and Dewey WC: Thermal dose determination in cancer therapy. Int J Radiat Oncol Biol Phys. 10:787–800. 1984. View Article : Google Scholar : PubMed/NCBI | |
|
Maguire PD, Samulski TV, Prosnitz LR, Jones EL, Rosner GL, Powers B, Layfield LW, Brizel DM, Scully SP, Harrelson JM, et al: A phase II trial testing the thermal dose parameter CEM43 degrees T90 as a predictor of response in soft tissue sarcomas treated with pre-operative thermoradiotherapy. Int J Hyperthermia. 17:283–290. 2001. View Article : Google Scholar : PubMed/NCBI | |
|
Dewhirst MW, Vujaskovic Z, Jones E and Thrall D: Re-setting the biologic rationale for thermal therapy. Int J Hyperthermia. 21:779–790. 2005. View Article : Google Scholar : PubMed/NCBI | |
|
de Bruijne M, van der Holt B, van Rhoon GC and van der Zee J: Evaluation of CEM43 degrees CT90 thermal dose in superficial hyperthermia: A retrospective analysis. Strahlenther Onkol. 186:436–443. 2010. View Article : Google Scholar : PubMed/NCBI | |
|
Assi H: A New CEM43 Thermal Dose Model Based On Vogel - Tammann-Fulcher Behaviour in Thermal Damage Processes. Ryerson University; Toronto: 2009 | |
|
Thrall DE, Prescott DM, Samulski TV, Rosner GL, Denman DL, Legorreta RL, Dodge RK, Page RL, Cline JM, Lee J, et al: Radiation plus local hyperthermia versus radiation plus the combination of local and whole-body hyperthermia in canine sarcomas. Int J Radiat Oncol Biol Phys. 34:1087–1096. 1996. View Article : Google Scholar : PubMed/NCBI | |
|
Franckena M: Hyperthermia for the Treatment of Locally Advanced Cervix Cancer. Erasmus University; Rotterdam: 2010 | |
|
Van der Zee J: Radiotherapy and Hyperthermia in Cervical Cancer, ESTRO/TMH, presentation. Mumbai: March 2–2005 | |
|
Franckena M, Fatehi D, de Bruijne M, Canters RA, van Norden Y, Mens JW, van Rhoon GC and van der Zee J: Hyperthermia dose- effect relationship in 420 patients with cervical cancer treated with combined radiotherapy and hyperthermia. Eur J Cancer. 45:1969–1978. 2009. View Article : Google Scholar : PubMed/NCBI | |
|
Schooneveldt G, Bakker A, Balidemaj E, Chopra R, Crezee J, Geijsen ED, Hartmann J, Hulshof MC, Kok HP, Paulides MM, et al: Thermal dosimetry for bladder hyperthermia treatment. An overview Int J Hyperthermia. 32:417–433. 2016. View Article : Google Scholar | |
|
Fotopoulou C, Cho CH, Kraetschell R, Gellermann J, Wust P, Lichtenegger W and Sehouli J: Sehoul: Regional abdominal hyperthermia combined with systemic chemotherapy for the treatment of patients with ovarian cancer relapse: Results of a pilot study. Int J Hyp. 26:118–126. 2009. View Article : Google Scholar | |
|
Thrall DE, LaRue SM, Yu D, Samulski T, Sanders L, Case B, Rosner G, Azuma C, Poulson J, Pruitt AF, et al: Thermal dose is related to duration of local control in canine sarcomas treated with thermoradiotherapy. Clin Cancer Res. 11:5206–5214. 2005. View Article : Google Scholar : PubMed/NCBI | |
|
Blank M and Goodman R: Electromagnetic fields stress living cells. Pathophysiology. 16:71–78. 2009. View Article : Google Scholar : PubMed/NCBI | |
|
Giuliani L and Soffritti M: Non-Thermal Effects And Mechanisms Of Interaction Between Electromagnetic Fields and Living Matter. An ICEMS Monograph; National Institute for the Study and Control of Cancer and Environmental Diseases ‘Bernardino Ramazzini’. 5. Fidenza Publication; Bologna: 2010 | |
|
Vincze G and Szasz A: Critical analysis of the thermodynamics of reaction kinetics. J Adv Phys. 10:2538–2559. 2015. View Article : Google Scholar | |
|
Dewey WC: Arrhenius relationships from the molecule and cell to the clinic. Int J Hyperthermia. 25:3–20. 2009. View Article : Google Scholar : PubMed/NCBI | |
|
Lindholm CE: Hyperthermia and Radiotherapy PhD Thesis. Lund University; Malmö: 1992 | |
|
Hafström L, Rudenstam CM, Blomquist E, Ingvar C, Jönsson PE, Lagerlöf B, Lindholm C, Ringborg U, Westman G and Ostrup L; Swedish Melanoma Study Group: Regional hyperthermic perfusion with melphalan after surgery for recurrent malignant melanoma of the extremities. J Clin Oncol. 9:2091–2094. 1991. View Article : Google Scholar | |
|
Bhowmick P, Coad JE, Bhowmick S, Pryor JL, Larson T, De La Rosette J and Bischof JC: In vitro assessment of the efficacy of thermal therapy in human benign prostatic hyperplasia. Int J Hyperthermia. 20:421–439. 2004. View Article : Google Scholar : PubMed/NCBI | |
|
Urano M and Douple E: Chemopotentiation by Hyperthermia. Hyperthermia in Oncology. 4. VSP Utrecht; Tokyo: pp. 1731994 | |
|
Digel I, Maggakis-Kelemen Ch, Zerlin KF, Linder P, Kasischke N, Kayser P, Porst D, Temiz Artmann A and Artmann GM: Body temperature-related structural transitions of monotremal and human hemoglobin. Biophys J. 91:3014–3021. 2006. View Article : Google Scholar : PubMed/NCBI | |
|
Lindegaard JC: Winner of the Lund Science Award 1992 Thermosensitization induced by step-down heating. A review on heat-induced sensitization to hyperthermia alone or hyperthermia combined with radiation. Int J Hyperthermia. 8:561–586. 1992. View Article : Google Scholar : PubMed/NCBI | |
|
van Rijn J, van den Berg J, Wiegant FA and van Wijk R: Time-temperature relationships for step-down heating in normal and thermotolerant cells. Int J Hyperthermia. 10:643–652. 1994. View Article : Google Scholar : PubMed/NCBI | |
|
Henle KJ and RotiRoti JL: Response of cultured mammalian cells to hyperthermia. Hyperthermia and Oncology. Urano M and Douple E: 1. VSP, Utrecht; Tokyo: pp. 57–82. 1988 | |
|
Konings AW: Interaction of heat and radiation in vitro and in vivo. Thermo-radiotherapy and Thermo-chemotherapy Biology, Physiology and Physics. 1. Seegenschmiedt MH, Fessenden P and Vernon CC: Springer Verlag; Berlin: pp. 89–102. 1995 | |
|
Hasegawa T, Gu YH, Takahashi T, Hasegawa T and Yamamoto I: Enhancement of hyperthermic effects using rapid heating. Thermotherapy for Neoplasia, Inflammation, and Pain. Kosaka M, Sugahara T and Schmidt KL: Springer Verlag; Tokyo-Berlin: pp. 439–444. 2001, View Article : Google Scholar | |
|
Biosca JA, Travers F and Barman TE: A jump in an Arrhenius plot can be the consequence of a phase transition. The binding of ATP to myosin subfragment 1. FEBS Lett. 153:217–220. 1983. View Article : Google Scholar : PubMed/NCBI | |
|
Watson K, Bertoli E and Griffiths DE: Phase transitions in yeast mitochondrial membranes. The effect of temperature on the energies of activation of the respiratory enzymes o. Saccharomyces cerevisiae = Biochem J. 146:401–407. 1975. | |
|
Szigeti, GyP, Szasz O and Hegyi G: Personalised dosing of hyperthermia. J Cancer Diagn. 1:1072016. View Article : Google Scholar | |
|
Erdmann B, Lang J and Seebass M: Optimization of temperature distributions for regional hyperthermia based on a nonlinear heat transfer model. Ann NY Acad Sci. 858:36–46. 1998. View Article : Google Scholar | |
|
Vincze G, Szasz O and Szasz A: Generalization of the thermal dose of hyperthermia in oncology. Open J Biophys. 5:97–114. 2015. View Article : Google Scholar | |
|
Pearce JA: Comparative analysis of mathematical models of cell death and thermal damage processes. Int J Hyperthermia. 29:262–280. 2013. View Article : Google Scholar : PubMed/NCBI | |
|
Geiser F and McMurchie EJ: Arrhenius parameters of mitochondrial membrane respiratory enzymes in relation to thermoregulation in endotherms. J Comp Physiol B. 155:711–715. 1985. View Article : Google Scholar : PubMed/NCBI | |
|
Geiser F and McMurchie EJ: Differences in the thermotropic behavior of mitochondrial membrane respiratory enzymes from homeothermic and heterothermic endotherms. J Comp Physiol B. 155:125–133. 1984. View Article : Google Scholar | |
|
Oleson JR, Calderwood SK, Coughlin CT, Dewhirst MW, Gerweck LE, Gibbs FA Jr and Kapp DS: Biological and clinical aspects of hyperthermia in cancer therapy. Am J Clin Oncol. 11:368–380. 1988. View Article : Google Scholar : PubMed/NCBI | |
|
Laider KJ: The development of the Arrhenius equation. J Chem Educ. 61:494–498. 1984. View Article : Google Scholar | |
|
Gardiner WC Jr: Temperature dependence of bimolecular gas reaction rates. Acc Chem Res. 10:326–331. 1977. View Article : Google Scholar | |
|
Petrou AL, Roulia M and Tampouris K: The use of the Arrhenius equation in the study of deterioration and of cooking of foods - some scientific and pedagogic aspects. Chem Educ Res And Pract In Eur. 3:87–97. 2002. View Article : Google Scholar | |
|
Qin Z, Balasubramanian SK, Wolkers WF, Pearce JA and Bischof JC: Correlated parameter fit of arrhenius model for thermal denaturation of proteins and cells. Ann Biomed Eng. 42:2392–2404. 2014. View Article : Google Scholar : PubMed/NCBI | |
|
Whitney J, Carswell W and Rylander N: Arrhenius parameter determination as a function of heating method and cellular microenvironment based on spatial cell viability analysis. Int J Hyperthermia. 29:281–295. 2013. View Article : Google Scholar : PubMed/NCBI | |
|
Scalley ML and Baker D: Protein folding kinetics exhibit an Arrhenius temperature dependence when corrected for the temperature dependence of protein stability. Proc Natl Acad Sci USA. 94:10636–10640. 1997. View Article : Google Scholar : PubMed/NCBI | |
|
Abney JR and Owicki JC: Theories of protein-lipid and protein-protein interactions in membranes. Progress in Protein-Lipid Interactions. Watts A and de Pont JJ: 1. Elsevier Biomedical Press; Amsterdam: pp. 1–60. 1985 | |
|
Evans MG and Polanyi M: Some applications of the transition state method to the calculation of reaction velocities, especially in solution. Trans Faraday Soc. 31:875–894. 1935. View Article : Google Scholar | |
|
Eyring H: The activated complex in chemical reactions. J Chem Phys. 3:107–115. 1935. View Article : Google Scholar | |
|
Laidler KJ and King MC: The development of transition-state theory. J Phys Chem. 87:2657–2664. 1983. View Article : Google Scholar | |
|
McRae DA and Esrick MA: Changes in electrical impedance of skeletal muscle measured during hyperthermia. Int J Hyperthermia. 9:247–261. 1993. View Article : Google Scholar : PubMed/NCBI | |
|
Pearce JA: Improving accuracy in arrhenius models of cell death: Adding a temperature-dependent time delay. Transactions of the ASME. J Biomech Eng. 137:1210062015. View Article : Google Scholar | |
|
Eyring H, Gershinowitz H and Sun CE: The absolute rate of homogeneous atomic reactions. J Chem Phys. 3:786–796. 1935. View Article : Google Scholar | |
|
Wynne-Jones WF and Eyring H: The absolute rate of reactions in condensed phases. J Chem Phys. 3:492–502. 1935. View Article : Google Scholar | |
|
Eyring H and Polanyi M: Über Einfache Gasreaktionen. Z Phys Chem B. 12:279–311. 1931.In German. | |
|
Eyring H and Stearn AE: The application of the theory of absolute reaction rates to proteins. Chem Rev. 24:253–270. 1939. View Article : Google Scholar | |
|
Crawford BL Jr: Quantum Chemistry. Eyring Henry, Walter John and Kimball George E.: J Phys Chem. 49:168. 1945. View Article : Google Scholar | |
|
Szasz A, Szasz N and Szasz O: Oncothermia - Principles principles and practices. Springer; Science, Heidelberg: 2010 | |
|
Wright NT: On a relationship between the Arrhenius parameters from thermal damage studies. J Biomech Eng. 125:300–304. 2003. View Article : Google Scholar : PubMed/NCBI | |
|
He X: Thermostability of biological systems: Fundamentals, challenges, and quantification. Open Biomed Eng J. 5:47–73. 2011. View Article : Google Scholar : PubMed/NCBI | |
|
He X and Bischof JC: Quantification of temperature and injury response in thermal therapy and cryosurgery. Crit Rev Biomed Eng. 31:355–422. 2003. View Article : Google Scholar | |
|
Jacques SL: Ratio of entropy to enthalpy in thermal transitions in biological tissues. J Biomed Opt. 11:0411082006. View Article : Google Scholar : PubMed/NCBI | |
|
Rosenberg B, Kemeny G, Switzer RC and Hamilton TC: Quantitative evidence for protein denaturation as the cause of thermal death. Nature. 232:471–473. 1971. View Article : Google Scholar : PubMed/NCBI | |
|
Low PS, Bada JL and Somero GN: Temperature adaptation of enzymes: Roles of the free energy, the enthalpy, and the entropy of activation. Proc Natl Acad Sci USA. 70:430–432. 1973. View Article : Google Scholar : PubMed/NCBI | |
|
Pearce JA: Thermal dose models: Irreversible alterations in tissues. Physics of Thermal Therapy, Fundamentals and Clinical Applications. Moros EG: CRC Press Taylor and Francis Group; Boca Raton, FL: 2013 | |
|
Lee SY, Kim JH, Han YH and Cho DH: The effect of modulated electro-hyperthermia on temperature and blood flow in human cervical carcinoma. Int J Hyperthermia. 34:953–960. 2018. View Article : Google Scholar : PubMed/NCBI | |
|
Lee SY, Lee NR, Cho DH and Kim JS: Treatment outcome analysis of chemotherapy combined with modulated electro- hyperthermia compared with chemotherapy alone for recurrent cervical cancer, following irradiation. Oncol Lett. 14:73–78. 2017. View Article : Google Scholar : PubMed/NCBI | |
|
Szasz O, Szasz MA, Minnaar C and Szasz A: Heating preciosity: Trends in modern oncological hyperthermia. Open J Biophys. 7:116–144. 2017. View Article : Google Scholar | |
|
Szent-Gyoergyi A: Cell division and cancer. Science. 149:34–37. 1965. View Article : Google Scholar : PubMed/NCBI | |
|
Davies PC, Demetrius L and Tuszynski JA: Cancer as a dynamical phase transition. Theor Biol Med Model. 8:302011. View Article : Google Scholar : PubMed/NCBI | |
|
Alfarouk KO, Shayoub ME, Muddathir AK, Elhassan GO and Bashir AH: Evolution of tumor metabolism might reflect carcinogenesis as a reverse evolution process (dismantling of multi-cellularity). Cancers (Basel). 3:3002–3017. 2011. View Article : Google Scholar | |
|
Greaves M: Evolutionary determinants of cancer. Cancer Discov. 5:806–820. 2015. View Article : Google Scholar : PubMed/NCBI | |
|
Bussey KJ, Cisneros LH, Lineweaver CH and Davies PC: Ancestral gene regulatory networks drive cancer. Proc Natl Acad Sci USA. 114:6160–6162. 2015. View Article : Google Scholar | |
|
Davies PC and Lineweaver CH: Cancer tumors as Metazoa 1.0: Tapping genes of ancient ancestors. Phys Biol. 8:0150012011. View Article : Google Scholar : PubMed/NCBI | |
|
Warburg O: Oxygen, the Creator of Differentiation, Biochemical Energetics. Academic Press; New York NY: 1966, Warburg O: The Prime Cause and Prevention of Cancer, Revised lecture at the meeting of the Nobel-Laureates on June 30, 1966 at Lindau, Lake Constance, Germany, 1966. | |
|
Oehr P, Biersack HJ and Coleman RE: PET and PET-CT in Oncology. Springer Verlag; Berlin, Heidelberg: 2004, View Article : Google Scholar | |
|
Mikac U, Demsar F, Beravs K and Sersa I: Magnetic resonance imaging of alternating electric currents. Magn Reson Imaging. 19:845–856. 2001. View Article : Google Scholar : PubMed/NCBI | |
|
Szasz A, Vincze Gy, Szasz O and Szasz N: An energy analysis of extracellular hyperthermia. Electromagn Biol Med. 22:103–115. 2003. View Article : Google Scholar | |
|
Szentgyorgyi A: Bioelectronics: A Study on Cellular Regulations, Defense and Cancer. Academic Press; London: pp. 1968 | |
|
Foster KR and Schepps JL: Dielectric properties of tumor and normal tissues at radio through microwave frequencies. J Microw Power. 16:107–119. 1981. View Article : Google Scholar : PubMed/NCBI | |
|
Blad B and Baldetorp B: Impedance spectra of tumour tissue in comparison with normal tissue; a possible clinical application for electric impedance tomography. Physiol Meas. 17(Suppl 4A): A105–A115. 1996. View Article : Google Scholar | |
|
Babaeizadeh S, Brooks DH and Isaacson D: 3-D electrical impedance tomography for piecewise constant domains with known internal boundaries. IEEE Trans Biomed Eng. 54:2–10. 2007. View Article : Google Scholar : PubMed/NCBI | |
|
Scholz B and Anderson R: On electrical impedance scanning - Principles and simulations. Electromedica. 68:35–44. 2000. | |
|
Musha T and Sawada Y: Physics of the Living Atate. IOS Press; Amsterdam: 1994 | |
|
West BJ: Fractal Physiology and Chaos in Medicine. World Scientific; London: 1990, View Article : Google Scholar | |
|
Lovelady DC, Richmond TC, Maggi AN, Lo CM and Rabson DA: Distinguishing cancerous from noncancerous cells through analysis of electrical noise. Phys Rev E Stat Nonlin Soft Matter Phys. 76:0419082007. View Article : Google Scholar : PubMed/NCBI | |
|
Szasz O, Andocs G and Meggyeshazi N: Modulation effect in oncothermia. Hindawi Publishing Corporation Conference Papers in Medicine. 2013.e3956782013. | |
|
Szasz A, Szasz N and Szasz O: Oncothermia - Principles and practices. Springer Science; Heidelberg: pp. 2202010 | |
|
Yang KL, Huang CC, Chi MS, Chiang HC, Wang YS, Andocs G, et al: In vitro comparison of conventional hyper-thermia and modulated electro-hyperthermia. Oncotarget. 7:84082–84092. 2016.PubMed/NCBI | |
|
Pethig R: Dielectric properties of biological materials: Biophysical and medical applications. IEEE Transactions on Electrical Insulation. E1–19. 453–474. 1984. | |
|
Schwan HP: Determination of biological impedances. Physical Techniques in Biological Research. 6. Academic Press; New York NY: pp. 323–406. 1963 | |
|
Pennock BE and Schwan HP: Further observations on the electrical properties of hemoglobin-bound water. J Phys Chem. 73:2600–2610. 1969. View Article : Google Scholar : PubMed/NCBI | |
|
Szasz O and Szasz A: Oncothermia - nano-heating paradigm. J Cancer Sci Ther. 6:42014. View Article : Google Scholar | |
|
Vincze Gy, Szigeti Gy, Andocs G and Szasz A: Nanoheating without artificial nanoparticles. Biol Med (Aligarh). 7:2492015. | |
|
Szasz A: Electromagnetic effects in nanoscale range. Cellular Response to Physical Stress and Therapeutic Applications. Shimizu T and Kondo T: Nova Science Publishers Inc.; Hauppauge, NY: 2013 | |
|
Andocs G, Rehman MU, Zhao QL, Papp E, Kondo T and Szasz A: Nanoheating without artificial nanoparticles Part II. Experimental support of the nanoheating concept of the modulated electro- hyperthermia method, using U937 cell suspension model. Biol Med (Aligarh). 7:1–9. 2015. View Article : Google Scholar | |
|
Andocs G, Rehman MU, Zhao QL, Tabuchi Y, Kanamori M and Kondo T: Comparison of biological effects of modulated electro-hyperthermia and conventional heat treatment in human lymphoma U937 cells. Cell Death Discov. 2:160392016. View Article : Google Scholar : PubMed/NCBI | |
|
Andocs G, Renner H, Balogh L, Fonyad L, Jakab C and Szasz A: Strong synergy of heat and modulated electro-magnetic field in tumor cell killing, Study of HT29 xenograft tumors in a nude mice model. Strahlenther Onkol. 185:120–126. 2009. View Article : Google Scholar : PubMed/NCBI | |
|
Andocs G, Okamoto Y, Osaki T, Tsuka T, Imagawa T, Minami S, Balogh L, Meggyeshazi N and Szasz O: Oncothermia research at preclinical level. Hindawi Publishing Corporation Conference Papers in Medicine; 2013, pp. e2724672013 | |
|
Szasz A: Current status of oncothermia therapy for lung cancer. Korean J Thorac Cardiovasc Surg. 47:77–93. 2014. View Article : Google Scholar : PubMed/NCBI | |
|
Meggyeshazi N: Studies on modulated electrohyperthermia induced tumor cell death in a colorectal carcinoma model. PhD Theses. Pathological Sciences Doctoral School, Semmelweis University; Budapest: 2015 | |
|
Meggyeshazi N, Andocs G, Balogh L, Balla P, Kiszner G, Teleki I, Jeney A and Krenacs T: DNA fragmentation and caspase-independent programmed cell death by modulated electrohyperthermia. Strahlenther Onkol. 190:815–822. 2014. View Article : Google Scholar : PubMed/NCBI | |
|
Andocs G, Meggyeshazi N, Balogh L, Spisak S, Maros ME, Balla P, Kiszner G, Teleki I, Kovago C and Krenacs T: Upregulation of heat shock proteins and the promotion of damage- associated molecular pattern signals in a colorectal cancer model by modulated electrohyperthermia. Cell Stress Chaperones. 20:37–46. 2015. View Article : Google Scholar | |
|
Qin W, Akutsu Y, Andocs G, Suganami A, Hu X, Yusup G, Komatsu-Akimoto A, Hoshino I, Hanari N, Mori M, et al: Modulated electro-hyperthermia enhances dendritic cell therapy through an abscopal effect in mice. Oncol Rep. 32:2373–2379. 2014. View Article : Google Scholar : PubMed/NCBI | |
|
Tsang YW, Huang CC, Yang KL, Chi MS, Chiang HC, Wang YS, Andocs G, Szasz A, Li WT and Chi KH: Improving immunological tumor microenvironment using electro-hyperthermia followed by dendritic cell immunotherapy. BMC Cancer. 15:7082015. View Article : Google Scholar : PubMed/NCBI | |
|
Szasz O and Szasz A: Heating, efficacy and dose of local hyperthermia. Open J Biophys. 6:10–18. 2016. View Article : Google Scholar | |
|
Elmore S: Apoptosis: A review of programmed cell death. Toxicol Pathol. 35:495–516. 2007. View Article : Google Scholar : PubMed/NCBI | |
|
Chang LK, Putcha GV, Deshmukh M and Johnson EM Jr: Mitochondrial involvement in the point of no return in neuronal apoptosis. Biochimie. 84:223–231. 2002. View Article : Google Scholar : PubMed/NCBI | |
|
Langdon BB, Kastantin M and Schwartz DK: Apparent activation energies associated with protein dynamics on hydrophobic and hydrophilic surfaces. Biophys J. 102:2625–2633. 2012. View Article : Google Scholar : PubMed/NCBI | |
|
Melnick RL, Hanson RM and Morris HP: Membranous effects on adenosine triphosphatase activities of mitochondria from rat liver and Morris hepatoma 3924A. Cancer Res. 37:4395–4399. 1977.PubMed/NCBI | |
|
Kushnareva Y, Andreyev AY, Kuwana T and Newmeyer DD: Bax activation initiates the assembly of a multimeric catalyst that facilitates Bax pore formation in mitochondrial outer membranes. PLoS Biol. 10:e10013942012. View Article : Google Scholar : PubMed/NCBI | |
|
Pouliquen D, Bellot G, Guihard G, Fichet P, Meflah K and Vallette FM: Mitochondrial membrane permeabilization produced by PTP, Bax and apoptosis: A 1H-NMR relaxation study. Cell Death Differ. 13:301–310. 2006. View Article : Google Scholar | |
|
De Virville JD, Cantrel C, Bousquet AL, Hoffelt M, Tenreiro AM, Vaz Pinto V, Arrabaca JD, Caiveau O, Moreau F and Zachowski A: Homeoviscous and functional adaptations of mitochondrial membranes to growth temperature in soybean seedlings. Plant Cell Environ. 25:1289–1297. 2002. View Article : Google Scholar | |
|
Lenaz G, Sechi AM, Parenti-Castelli G, Landi L and Bertoli E: Activation energies of different mitochondrial enzymes: Breaks in Arrhenius plots of membrane-bound enzymes occur at different temperatures. Biochem Biophys Res Commun. 49:536–542. 1972. View Article : Google Scholar : PubMed/NCBI | |
|
Lee M and Vasioukhin V: Cell polarity and cancer - cell and tissue polarity as a non-canonical tumor suppressor. J Cell Sci. 121:1141–1150. 2008. View Article : Google Scholar : PubMed/NCBI | |
|
Litovitz TA, Krause D, Penafiel M, Elson EC and Mullins JM: The role of coherence time in the effect of microwaves on ornithine decarboxylase activity. Bioelectromagnetics. 14:395–403. 1993. View Article : Google Scholar : PubMed/NCBI | |
|
Kim EK and Choi EJ: Pathological roles of MAPK signaling pathways in human diseases. Biochim Biophys Acta. 1802.396–405. 2010. | |
|
Leszczynski D, Joenväärä S, Reivinen J and Kuokka R: Non-thermal activation of the hsp27/p38MAPK stress pathway by mobile phone radiation in human endothelial cells: Molecular mechanism for cancer- and blood-brain barrier-related effects. Differentiation. 70:120–129. 2002. View Article : Google Scholar : PubMed/NCBI | |
|
Cuesta-López S, Errami J, Falo F and Peyrard M: Can we model DNA at the mesoscale. J Biol Phys. 31:273–301. 2005. View Article : Google Scholar | |
|
Takahashi A, Matsumoto H, Nagayama K, Kitano M, Hirose S, Tanaka H, Mori E, Yamakawa N, Yasumoto J, Yuki K, et al: Evidence for the involvement of double-strand breaks in heat- induced cell killing. Cancer Res. 64:8839–8845. 2004. View Article : Google Scholar : PubMed/NCBI | |
|
Kim JK, Prasad B and Kim S: Temperature mapping and thermal dose calculation in combined radiation therapy and 1356 MHz radiofrequency hyperthermia for tumor treatment Proc SPIE 10047. Opt Methods Tumor Treat Detect Mech Tech Photodynamic Ther. 26:10047182017. | |
|
Szasz O, Andocs G and Meggyeshazi N: Oncothermia as Personalized Treatment Option. Hindawi Publishing Corporation Conference Papers in Medicine 2013. e29413642013. | |
|
Fowler JF: The first James Kirk memorial lecture. What next in fractionated radiotherapy. Br J Cancer. 6:285–300. 1984. | |
|
Wang JZ, Li XA, D'Souza WD and Stewart RD: Impact of prolonged fraction delivery times on tumor control: A note of caution for intensity-modulated radiation therapy (IMRT). Int J Radiat Oncol Biol Phys. 57:543–552. 2003. View Article : Google Scholar : PubMed/NCBI | |
|
Angus SD and Piotrowska MJ: A matter of timing: identifying significant multi-dose radiotherapy improvements by numerical simulation and genetic algorithm search. PLoS One. 9:e1140982014. View Article : Google Scholar : PubMed/NCBI | |
|
Andocs G, Okamoto Y, Kawamoto K, Osaki T, Tsuka T, Imagawa T, Miniami S, Balogh L, Meggysházi N and Szasz O: Oncothermia basic research at in vivo level The first results in Japan. Hindawi Publishing Corporation Conference Papers in Medicine 2013. e1973282013. | |
|
Kim W, Kim MS, Kim HJ, Lee E, Jeong JH, Park I, Jeong YK and Jang WI: Role of HIF-1α in response of tumors to a combination of hyperthermia and radiation in vivo. Int J Hyperthermia. 28:1–8. 2017. | |
|
Lee SY and Kim MG: The effect of modulated electro-hyperthermia on the pharmacokinetic properties of nefopam in healthy volunteers: A randomised, single-dose, crossover open-label study. Int J Hyperthermia. 31:869–874. 2015. View Article : Google Scholar : PubMed/NCBI | |
|
Meggyeshazi N, Andocs G and Krenacs T: Programmed cell death induced by modulated electro-hyperthermia. Hindawi Publishing Corporation Conference Papers in Medicine 2013. e187835.2013. |